Ellipse

The search term "Elliptical" redirects to this page; for the exercise machine, see Elliptical trainer.

In mathematics, an ellipse (from the Greek for absence) is the locus of points on a plane where the sum of the distances from any point on the curve to two fixed points is constant. The two fixed points are called foci (plural of focus).

An ellipse is a type of conic section: if a conical surface is cut with a plane which does not intersect the cone's base, the intersection of the cone and plane is an ellipse. For a short elementary proof of this, see Dandelin spheres.

Algebraically, an ellipse is a curve in the Cartesian plane defined by an equation of the form

Ax^{2}+Bxy+Cy^{2}+Dx+Ey+F=0

such that $B^{2}<4AC$ , where all of the coefficients are real, and where more than one solution, defining a pair of points (x, y) on the ellipse, exists.

An ellipse can be drawn with two pins, a loop of string, and a pencil. The pins are placed at the foci and the pins and pencil are enclosed inside the string. The pencil is placed on the paper inside the string, so the string is taut. The string will form a triangle. If the pencil is moved around so that the string stays taut, the sum of the distances from the pencil to the pins will remain constant, satisfying the definition of an ellipse.

The line segment that passes through the foci and terminates on the ellipse is called the major axis. The major axis is along the longest segment that passes through the ellipse. The line which passes through the center (halfway between the foci), at right angles to the major axis, is called the minor axis. A semimajor axis is one half the major axis: the line segment from the center, through a focus, and to the edge of the ellipse. Likewise, the semiminor axis is one half the minor axis.

If the two foci coincide, then the ellipse is a circle; in other words, a circle is a special case of an ellipse, one where the eccentricity is zero.

An ellipse centred at the origin can be viewed as the image of the unit circle under a linear map associated with a symmetric matrix $A=PDP^{T}$ , D being a diagonal matrix with the eigenvalues of A, both of which are real positive, along the main diagonal, and P being a real unitary matrix having as columns the eigenvectors of A. Then the axes of the ellipse will lie along the eigenvectors of A, and the eigenvalues are the lengths of the semimajor and semiminor axes.

An ellipse can be produced by multiplying the x coordinates of all points on a circle by a constant, without changing the y coordinates.

Parameterisation

y=b\,\sin \beta \,\!

where $\beta$ can be limited by the interval

0\leq \beta <2d

The following diagram shows an ellipse demonstrating the Pythagoras equation a² = b² + c² as a special case of the non-parametric equation above (x=0, y=b).

If an ellipse is not centered at the origin of an x-y coordinate system, but again has its major axis along the x-axis, it may be specified by the equation

{\frac {(x-h)^{2}}{a^{2}}}+{\frac {(y-k)^{2}}{b^{2}}}=1

where (h,k) is the center.

If an ellipse is not centered at the origin of an x-y coordinate system and has its major axis along the y-axis, it may be specified by the equation

{\frac {(y-k)^{2}}{a^{2}}}+{\frac {(x-h)^{2}}{b^{2}}}=1

where (h,k) is the center.

In polar coordinates, where the origin is one focus of the ellipse:

r={\frac {a(1-e^{2})}{1+e\cos(\theta )}}

A Gauss-mapped form:

\left({\frac {a^{2}\cos \phi }{\sqrt {a^{2}\cos ^{2}\phi +b^{2}\sin ^{2}\phi }}},{\frac {b^{2}\sin \phi }{\sqrt {a^{2}\cos ^{2}\phi +b^{2}\sin ^{2}\phi }}}\right)

has normal $(\cos \phi ,\sin \phi )$ . |

Eccentricity

The shape of an ellipse is usually expressed by a number called the eccentricity of the ellipse, conventionally denoted e (not to be confused with the mathematical constant e). The eccentricity is related to a and b by the statement

e={\sqrt {1-{\frac {b^{2}}{a^{2}}}}}

or where $c$ (the linear eccentricity of the ellipse) equals the distance from the center to either focus

e={\frac {c}{a}}

The eccentricity is a positive number less than 1, or 0 in the case of a circle. The greater the eccentricity is, the larger the ratio of a to b, and therefore the more elongated is the ellipse. The ellipse shown in the image below has an eccentricity of approximately 0.8733. The distance between the foci is 2ae.

Semi-latus rectum and polar coordinates

The semi-latus rectum of an ellipse, usually denoted $l\,\!$ (lowercase L), is the distance from a focus of the ellipse to the ellipse itself, measured along a line perpendicular to the major axis. It is related to $a\,\!$ and $b\,\!$ (the ellipse's semi-axes) by the formula $al=b^{2}\,\!$ or, if using the eccentricity, $l=a(1-e^{2})\,\!$ .

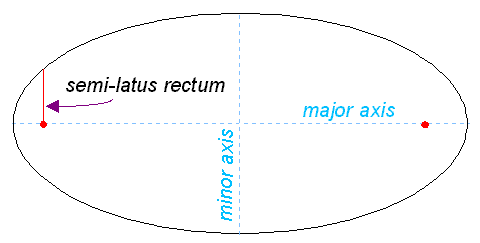
In polar coordinates, an ellipse with one focus at the origin and the other on the negative x-axis is given by the equation

r(1+e\cos \theta )=l\,\!

An ellipse can also be thought of as a projection of a circle: a circle on a plane at angle φ to the horizontal projected vertically onto a horizontal plane gives an ellipse of eccentricity sin φ, provided φ is not 90°.

Area

The area enclosed by an ellipse is πab, where 'a' and 'b' are the semimajor and semiminor axes and π is Archimedes's constant. In the case of a circle where a = b, the expression reduces to the familiar πa².

Circumference

The circumference of an ellipse is $4aE(e)$ , where the function E is the complete elliptic integral of the second kind.

The exact infinite series is:

C=2\pi a\left[{1-\left({1 \over 2}\right)^{2}e^{2}-\left({1\cdot 3 \over 2\cdot 4}\right)^{2}{e^{4} \over 3}-\left({1\cdot 3\cdot 5 \over 2\cdot 4\cdot 6}\right)^{2}{e^{6} \over 5}-\dots }\right]\!\,

Or:

C=2\pi a\sum _{n=0}^{\infty }{\left\lbrace -\left[\prod _{m=1}^{n}\left({2m-1 \over 2m}\right)\right]^{2}{e^{2n} \over 2n-1}\right\rbrace }

A good approximation is Ramanujan's:

C\approx \pi \left[3(a+b)-{\sqrt {(3a+b)(a+3b)}}\right]\!\,

which can also be written as:

C\approx \pi a\left[3(1+{\sqrt {1-e^{2}}})-{\sqrt {(3+{\sqrt {1-e^{2}}})(1+3{\sqrt {1-e^{2}}})}}\right]\!\,

More generally, the arc length of a portion of the circumference, as a function of the angle subtended, is given by an incomplete elliptic integral. The inverse function, the angle subtended as a function of the arc length, is given by the elliptic functions.

Stretching and projection

An ellipse may be uniformly stretched along any axis, in or out of the plane of the ellipse, and it will still be an ellipse. The stretched ellipse will have different properties (perhaps changed eccentricity and semi-major axis length, for instance), but it will still be an ellipse (or a degenerate ellipse: a circle or a line). Similarly, any oblique projection onto a plane results in a conic section. If the projection is a closed curve on the plane, then the curve is an ellipse or a degenerate ellipse.

Reflection property

Assume an elliptic mirror with a light source at one of the foci. Then all rays are reflected to a single point — the second focus. Since no other curve has such a property, it can be used as an alternative definition of an ellipse.

Sound waves are reflected in a similar way, so in a large elliptical room a person standing at one focus can hear a person standing at another focus remarkably well. Such a room is called a whisper chamber. Examples are the National Statuary Hall Collection at the U.S. Capitol (where John Quincy Adams is said to have used this property for eavesdropping on political matters), at an exhibit on sound at the Museum of Science and Industry in Chicago, in front of the University of Illinois at Urbana-Champaign Foellinger Auditorium, and also at a side chamber of the Palace of Charles V, in the Alhambra.

Ellipses in physics

In 499, Indian astronomer Aryabhata discovered that the orbits of the planets around the sun are ellipses, and published his findings in his book, the Aryabhatiya [1].

In the 17th century, Johannes Kepler explained that the orbits along which the planets travel around the Sun are ellipses, which is Kepler's first law. Later, Isaac Newton explained this as a corollary of his law of universal gravitation.

More generally, in the gravitational two-body problem, if the two bodies are bound to each other (i.e., the total energy is negative), their orbits are similar ellipses with the common barycenter being one of the foci of each ellipse. Interestingly, the orbit of either body in the reference frame of the other is also an ellipse, with the other body at one focus.

The general solution for a harmonic oscillator in two or more dimensions is also an ellipse, but this time with the origin of the force located at the center of the ellipse.

Albert Einstein also used the ellipse to prove his theory of relativity by using an elliptical shaped mass.

Ellipses in computer graphics

Drawing an ellipse is a common graphics primitive in standard display libraries, such as the Macintosh QuickDraw API and the Windows Graphics Device Interface (GDI). Often such libraries are limited and can only draw an ellipse with either the major axis or the minor axis horizontal. Jack Bresenham at IBM is most famous for the invention of 2D drawing primitives, including line and circle drawing, using only fast integer operations such as addition and branch on carry bit. An efficient generalization to draw ellipses was invented in 1984 by Jerry Van Aken (IEEE CG&A, Sept. 1984). A more challenging task is to perform these drawing operations with antialiasing, to create a smooth-looking curve. Here is an example of 2D graphics code for the ellipse in C:

/* x1 - x coordinate of the most left point of the ellipse
   x2 - x of the most right point of the ellipse
   y1 - y of the lowest point of ellipse
   y2 - y of the highest point of ellipse
*/
void _ellipse(int x1, int y1, int x2, int y2)
{
   double t, a, b, tinc, centx, centy;
   a = fabs(.5 * (double)(x2 - x1));
   b = fabs(.5 * (double)(y2 - y1));
   tinc = PI * 2 / (a + b);
   centx = (double)((x1 + x2) + .5) * .5;
   centy = (double)((y1 + y2) + .5) * .5;

   _moveto(centx + a, centy);
   for(t = 0; t < PI * 2; t += tinc)
      _lineto(centx + a * cos(t), centy - b * sin(t));
}

External Links

Ellipse & Hyperbola Construction - An interactive sketch showing how to trace the curves of the ellipse and hyperbola. (Requires Java.)
Ellipse Construction - Another interactive sketch, this time showing a different method of tracing the ellipse. (Requires Java.)
Graph Ellipses Online