Talk:CIE 1931 color space
| This article is written in American English, which has its own spelling conventions (color, defense, traveled) and some terms that are used in it may be different or absent from other varieties of English. According to the relevant style guide, this should not be changed without broad consensus. |
| This It is of interest to the following WikiProjects: | |||||||||||
| |||||||||||
Please add new topics at the bottom
[edit]Please add new topics at the bottom
Luminance
[edit]Can someone explain from where the relation Y = 0.2125R + 0.7154G + 0.0721B came from? The matrix equation says Y = (1/0.17697)(0.17697R + 0.81240G + 0.01063B). Am I missing something?-- 19:00, 26 July 2006 MM
15.4 LUMINANCE of sRGB
[edit]For CIE1931 xyY 2° the LUMINANCE is:
with:
For sRGB the LUMINANCE is:
with:
Y is given by the second colum of M matrix
Y = 0.2126r + 0.7155g + 0.0721b
n1 is the normalization to 1
Divide each term by the sum of the row.
Primaries
| coord. | x | y | z |
|---|---|---|---|
| Red | 0.64 | 0.33 | 0.03 |
| Green | 0.30 | 0.60 | 0.10 |
| Blue | 0.06 | 0.06 | 0.79
|
15.4 Exemple de Adobe RGB
[edit]
Il s'agit d'un système colorimétrique développé par Adobe en 1998[1]. Il propose un gamut élargi par rapport à l'espace sRGB.
Couleurs Rouge R 608nm Vert G 538nm Bleu B 468nm Blanc D65 Coordonnée x 0,640 0,210 0,150 0,3127 Coordonnée y 0,330 0,710 0,060 0,3290 Coefficient de luminance L 0,29734 0,62736 0,07529 total=1 Coef de luminance barycentrique L/y 0,29644 0,29071 0,41285 total=1
Calculs des coefficients de luminance barycentrique
On cherche les luminances barycentriques a,b,c (Y/y) des 3 primaires qui donnent le point W(D65) (x=0,3127,y=0,3290)(z=0,3590)br/>
a=Lr/yr b=Lg/yg c=Lb/yb
Les formules donnant le barycentre des 3 primaires sont :
x=0,64 a+0,21 b+0,15 c=0,3127
y=0,33 a+0,71 b+0,06 c=0,3290
a+b+c=1
La résolution de ces 3 équations à 3 inconnues donne :
a=0,889332/3
b=0,872124/3
c=1,238544/3
yr*a=0,33*a=k*0,293479490572279/3
yg*b=0,71*b=k*0,619207839894145/3
yb*c=0,06*c=k*0,074312669533576/3
S=yr*a+yg*b+yb*c=k*0,987/3
yr*a/S=0,29734497=Lr
yg*b/S=0,62736356=Lg
yb*c/S=0,07529145=Lb
Ce sont bien les valeurs données par Adobe RGB (arrondies à 5 décimales)
La luminance visuelle du blanc sur un écran de référence est de 160,00 cd·m-2 (X = 152,07=486,32x, Y = 160,00=486,32y, Z = 174.25=486,32z)
15.5 Exemple de sRGB
[edit]Couleurs Rouge Vert Bleu Blanc Coordonnée x 0,64 0,3 0,15 0,3127 Coordonnée y 0,33 0,6 0,06 0,3290 Coefficient de luminance L 0,2126 0,62736 0,07529 total=1 Coef de luminance barycentrique L/y 0,2396 0,3535 0,4069 total=1
Vérification des coefficients de luminance barycentrique
Les luminances barycentriques a,b,c (Y/y) des 3 primaires donnent le point W(D65) (x=0,3127,y=0,3290)(z=0,3590)br/>
a=k*Lr/yr b=k*Lg/yg c=k*Lb/yb
a+b+c=1 après normalisation à l'unité
On vérifie les formules donnant le barycentre des 3 primaires :
x=0,64a+0,3b+0,15c=0,3127
y=0,33a+0,6b+0,06c=0,3290
— Preceding unsigned comment added by Bercier (talk • contribs) 15:34, 18 June 2020 (UTC)
Sorry for many mistakes
Bercier (talk) 10:25, 11 April 2013 (UTC) Bercier (talk) 09:53, 28 June 2013 (UTC)
Grassmann's Laws
[edit]If you look at the biography of Grassmann, you'll see it mentions his color work. The law comes, I believe, from his article "Theory of compound colors", Philosophical Magazine 4 (7), 1854, 254-264. You can cross-check in a few library catalogues under, e.g. Sources of color science, ed. David L. MacAdam, MIT Press [1970]. --Macrakis 23:35, 11 July 2005 (UTC)
This section should follow the section "Construction of the CIE XYZ color space from the Wright–Guild data" , as it discusses a simple special case of the CIE XYZ color spaces that are possible. MxBuck (talk)
Why is the bottom flat?
[edit]The outer contour iof the CIE figure lists wavelengths (from 380 to 700 nanometers), but what are the wavelengths of the colors along the straight line from 380 to 700?
How do they fit in, and why is that line straight?
- The wavelengths along the straight line at the bottom are called "non-spectral colors". They are colors which no monochromatic beam can match, they must be made up of a combination of two(only 2 and no more:the min of blue 380nm and the max of red 800nm) monochromatic beams. The outer contour is called the "spectral locus" and contains all of the colors that a monochromatic beam can match. In order to be a physically possible light beam, any beam must be made up of one or more monochromatic beams (or an infinite number for a continuous spectrum.)
- A second fact to note is that if you pick two points inside the range of possible colors (the gamut), every point on a straight line between the two must also lie inside the gamut. That means that every point between the two far ends of the spectral locus must lie inside the gamut. However, if a point were to lie outside the line connecting the two ends, then you would be unable to choose two or more monochromatic beams to specify that point. That means such a point is impossible. PAR 15:31, 6 September 2006 (UTC)
- This is probably a silly question, but isn't purple a part of the spectrum? 86.5.107.158 03:08, 20 September 2006 (UTC)
- The straight line mentioned above is sometimes called the "line of purples". If we define any color on the straight line as a type of purple, then, no, there is no wavelength that corresponds to purple. The sensation of purple can only be realized by using a combination of 2 monochromatic light sources(lower blue and bigger red). PAR 03:29, 20 September 2006 (UTC)
Bercier (talk) 13:13, 4 March 2013 (UTC)
- Purple is non spectral, but violet and indigo are spectral, crazy world right? — Preceding unsigned comment added by Da5nsy (talk • contribs) 22:06, 8 March 2013 (UTC)
mistake
[edit]The CIE XYZ color space was deliberately designed so that the Y parameter was a measure of the brightness of a color.
Should not be "Z parameter"?
- No, its the Y parameter. PAR 15:03, 6 September 2006 (UTC)
- because we see the most bright color in the medium of wavelenght, and Y is the present of this wave lenght, so it's the best choise. —Preceding unsigned comment added by 81.12.9.2 (talk) 20:31, 4 November 2007 (UTC)
- No; the CIE XYZ color space was most definitely not designed so that the Y coordinate (it's a coordinate, not a parameter) is the measure of brightness. The coordinate Y was assigned to the luminance of a color, which is a physical, not a perceptual (like brightness) attribute. It was believed that luminance was a linear combination of cone fundamentals, and the objection to existing color matching functions, such as König's, was that could only be approximated by a linear combination of color matching functions. See Wright's anniversary paper, for example. In any case, brightness and luminance are two different, but related things, just as sound pressure and loudness are. Hope this helps! Lovibond (talk) 01:34, 17 January 2012 (UTC)
chromaticity diagram
[edit]Is the xy chromaticity diamgram on page 2 plotted at a fixed brightness? Could one display any number of such diagrams with different brightnesses values or is this diagram always plotted at some special brightness?
- If you click on the diagram, it will take you to the diagram page. There is an explanation there of how the diagram was generated. PAR 01:54, 2 June 2006 (UTC)
The chromaticity diagram is not iso-luminous, because luminosity increases in Y and therefore in y. Why was the Maxwell triangle chosen over a plane of constant Y?
The diagram is simply a plot of x,y. Luminosity is related to y and so the top of the diagram must be more luminous than the base. Please distinguish between the theoretical diagram and the actual realisation. There is just one single theoretical diagram. Unfortunately it is not technically possible to display it on a screen nor is it possible to print it. Nevertheless the diagram in the article looks pretty good. The artist chose compromise colors very carefully and they were excellent choices. But they aren't, they cannot be, the actual colors - computer screens cannot produce spectral colours. A different artist might make different choices and so the diagram would look different but the underlying theoretical diagram would always remain the same.OrewaTel (talk) 02:07, 11 June 2019 (UTC)
normalization
[edit]Should
be instead something like
? As far as I understand, and are separately and empirically determined up to a scalar constant, so it doesn't make much sense to set one equal to the other. Jcreed 04:32, 29 September 2006 (UTC)
- That certainly looks right. I have changed it, and will check that it is in fact true. PAR 00:25, 20 October 2006 (UTC)
By definition :: 08:48, 13 April 2013 (UTC)
Purples outside the gamut of Wright and Guild's primaries ?
[edit]I think I understand how the blue-green out-of-gamut part of the XYZ color space can be derived from the Wright and Guild color matching functions (using negative values for red) but how does that work for the purples?
- I believe you would use negative values of both red and blue. PAR 00:25, 20 October 2006 (UTC)
- Thats wrong. Make it negative values of green. PAR 00:29, 20 October 2006 (UTC)
- Why aren't there negative values of green in the color matching functions as shown in the article? Those matching functions go down to 380nm, where they're outside of the Wright and Guild primaries' gamut.
- ProPhotoRGB also extends into near-UV spectrum (380nm); I believe recent 10bpp and higher(?) monitors are installing filters to prevent them being displayed, because there's nothing stopping the panel doing it. EdityMcEditorson (talk) 22:04, 27 September 2017 (UTC)
WRIGHT et ses 10 expérimentateurs ont utilisé les primaires B de 460 nm G de 530 nm et R de 650 nm. GUILD et ses 7 expérimentateurs ont utilisé les primaires B de 461,6 nm G de 542 nm et R de 627,7 nm. CIE1931 donne les valeurs des CMF de B 360 nm (proche des UV) à R 830 nm (proche des IR). V1924 est donnée de 360 nm à 830 nm. Le segment de droite 360 nm à 700 nm est le lieu des magentas, qui va du bleu (bleu-violet si on veut mais sombre au rouge noir). Les gamuts des écrans n'ont pas la possibilité d'utiliser ces longueurs d'onde extrêmes qui ne servent qu'à matérialiser le spectrum locus.
Bercier (talk) 16:01, 18 June 2020 (UTC)
Red response peak
[edit]"The 700 nm wavelength, which in 1931 was difficult to reproduce as a monochromatic beam, was chosen because it is at the peak of the eye's red response..."
If the supplied diagrams are correct, then the statement that 700 nm is at the peak of red response must be wrong. Maybe the authors meant "600 nm"? This is much closer to the peak.--WhAt 18:07, 26 December 2006 (UTC)
- Yes, that is an error, and I will fix it. To quote from "How the CIE 1931 Color-Matching Functions Were Derived from Wright–Guild Data" by Hugh S. Fairman, Michael H. Brill, and Henry Hemmendinger:
"Guild approached the problem from the viewpoint of a standardization engineer. In his mind, the adopted primaries had to be producible with national-standardizing-laboratory accuracy. The first two wavelengths were mercury excitation lines, and the last named wavelength occurred at a location in the human vision system where the hue of spectral lights was unchanging with wavelength. Slight inaccuracy in production of the wavelength of this spectral primary in a visual colorimeter, it was reasoned, would introduce no error at all."
700nm was choosen because it is at the end of the spectre,and so you haven't negative values.
Bercier (talk) 11:31, 6 February 2013 (UTC)
The Structure of The Article needs to be re-ordered?
[edit]Does the structure of the article needs to be re-ordered? The X,Y,Z comes from nowhere and then it is said to be the basis "on which many other color spaces are defined", but then it says XYZ is derived from RGB. So which one is more fundmental ? The XYZ space or the RGB?
Certainly the RGB is more fundmental! Because they want to eliminate the NEGATIVE value of Red component as a linear transform is taken, after which XYZ space forms. Therefore the article needs to talk about RGB first, followed by XYZ. After this, the normalization may be discussed!
The order of the article is totally upside-down!!!!
--Puekai 15:19, 19 Jan 2007(UTC)
- The RGB data is the statement of the direct measurements, yes. But the XYZ is just a reformulation of those results. Both carry the same content, its just that the RGB are the result of direct measurements, while XYZ is derived from those results. I don't think RGB is more fundamental, it is just closer to the actual experiments.
- The article is named "CIE 1931 color space" and is about the XYZ space. For someone who wants to learn about the CIE 1931 color space, they should not have to wade through two or three sections about RGB space first. Thats only for people interested in the foundations upon which the CIE space is built. PAR 15:35, 19 January 2007 (UTC)
- I have to disagree with you both. RGB is NOT more fundamental, and is NOT a result of direct measurements. There do not exist sets of spectral sensitivity curves that you can use in a sensor to directly measure RGB, precisely because of those negative regions in the RGB color matching function, which would mean your sensor would have to have negative sensitivities to some wavelengths. That's why you need to understand this stuff. But I'll review the article, because if it really does say that "XYZ is derived from RGB" then that needs to be fixed, since it's not true. Dicklyon 16:21, 19 January 2007 (UTC)
- Well, maybe it's right what it says about the history. The CIE RGB space may have been defined first, in terms of monochromatic primaries. However, that historical order doesn't make it more fundamental. Both spaces are based on the same experimental data, but with different goals for the properties of the color matching functions. I've ordered the referenced book so I can understand the history better. Dicklyon 16:31, 19 January 2007 (UTC)
- Yes, it needs to be clear that the "RGB" space here is not what is commonly called "RGB" space, it is a very particular set of measurements, and should probably be referred to as the "CIE RGB" space, or "Wright-Guild RGB" space, or something. PAR 16:46, 19 January 2007 (UTC)
- Indeed it is cleary identified as CIE RGB in the article. It's not a set of measurements, though, any more than CIE XYZ is; less, actually, since you can actually make filters and detectors that CAN measure X, Y, and Z, but you CANNOT for R, G, and B. Dicklyon 19:07, 19 January 2007 (UTC)
- Are you sure about this? It seems strange to me that you can measure X,Y, and Z, since of the three, only Y has a definition that is more or less physical, and it even depends on the experimental Luminosity function. I would say it's far more likely that the filters and detectors measure according to other values (possibly the way we do), and then transform it to X,Y,Z using whatever mathematical formula are needed. In this case, making "CIE RGB detectors" merely involves doing one more mathematical transformation.
- What I meant was that you can't measure R, G, and B by putting filters in front of photodetectors, since the sensitivity curves are negative at some wavelengths, and filter/detector systems can only have non-negative sensitivities at all wavelengths. The X, Y, and Z basis functions were specifically defined to be nonnegative, so that ALL chromaticities are inside the XYZ color triangle. You are correct that ANY set of sensitivities that span the same subspace of spectral space can be used and transformed by a 3x3 matrix multiply to XYZ or RGB. Dicklyon 04:33, 1 November 2007 (UTC)
- I also feel a bit uneasy about the statement that CIE RGB is not a result of measurements, since from what I understand, it was defined on the basis of these experiments (and to obtain negative values, they added the primary color to the test color). I'm also not sure about CIE XYZ not being derived from CIE RGB, since from what I understand, they first had a good look at their model of human vision in CIE RGB, then they decided they wanted to transform it linearly to something nicer, and it ended up being CIE XYZ. Ratfox 00:14, 1 November 2007 (UTC)
- If I understand your conjecture, it's that the RGB primaries are the same as what were used in the matching experiments. Maybe so. Let's look at sources and see. Dicklyon 04:33, 1 November 2007 (UTC)
- Here are some words about the Wright and Guild experiments and transformations to the RGB and XYZ basis functions. It says Wright used monochromatic primaries, but doesn't say what wavelengths. Dicklyon 04:40, 1 November 2007 (UTC)
- I strongly agree that the "RGB" space needs to be clearly differentiated from the 'common' RGB space. And I don't think writing "CIE RGB" is enough: the lay person could easily read this as saying that what they know as "RGB" is a 'short form' of "CIE RGB" (Like "McDonald's" versus "McDonald's Family Restaurants", the listing in the Australian telephone directory.). The section ought to start with a statement along the lines of "The CIE RGB colour space is one of many RGB colour spaces. It is based on the work of ...." and then go into the existing text.
- By the way, RGB color spaces says "Note that CIE 1931 (or CIE XYZ) is not an RGB color space." What's up with that? Completely contradicts this article!
- —DIV (128.250.204.118 04:47, 30 July 2007 (UTC))
- See if recent changes to both articles make more sense now. Feel free to make more suggestions for clarifications. Dicklyon 06:04, 30 July 2007 (UTC)
- I agree. This article doesn't explain things properly. It should be rewritten in such a way that anyone struggling in a course about this topic, after they study this article carefully would know how to answer any test question that relates any color to any color space they were taught about in the course. Blackbombchu (talk) 00:59, 2 May 2015 (UTC)
R,G, and B are never defined
[edit]I propose that these three variables be defined explicitly on this page. I know that you can find them out by clicking on the Grassman's law link, but it is rather awkward. That article is so short, why not just include the whole thing here? There's already a section heading for it. Bababoef 08:58, 7 April 2007 (UTC)
- OK, I did it, but I'm not totally comfortable having the overline{r} notation for both the normalized color matching functions and the scaled ones. Is there any ohter symbol people use to distinguish these? Dicklyon 16:22, 7 April 2007 (UTC)
Er, strictly speaking all of the functions are "scaled", aren't they? Even for the normalized color matching functions the normalization constant is not defined. (Is this the way it is in the literature?) I assume you mean scaled for luminance vs radiant pwr? I am not familiar with the notation in the literature but you are right, there is clearly a notation problem here. Perhaps the simplest way to resolve this is to define R,G, and B in the following manner:
I think this helps make everything more explicit while making the notation consistent and showing that the color spaces are defined in terms of radiant power. (Assuming i did everything right here)
On a related note, why do the ratios have differing numbers of significant figures? For example, for r:g:b = 1:4.5907:0.0601 the r:g ratio has 5 sig figs, the r:b ratio has 3 sig figs, and the g:b ratio has 3 sig figs. Shouldn't they all have the same number of significant figures since this is a color standard and can be defined to arbitrary precision? Bababoef 20:07, 7 April 2007 (UTC)
- I'm not sure picking the numbers for "source radiant power" means anything that I can understand. It's possible that no scale factor is actually the right thing; it would give a "neutral" result for the "E" (equal spectrum) illuminant, looks like. I'm not really very familiar with this space, but I have a book I can consult... Dicklyon 23:40, 7 April 2007 (UTC)
It has to be the radiant power curves. Otherwise you wouldn't integrate them with the "spectral power distribution", you'd integrate them with some luminous distribution. Bababoef 00:14, 8 April 2007 (UTC)
- Oh, I see what it is now; not what you're thinking, I believe; in all cases they are weights to be applied to the specral radiant power distribution. Those are the scale factors you need to convert from the RGB variables to the luminances or radiant powers of the three defined monochromatic primaries. Therefore, those scalings come after the RGB definition; we can use the normalized functions (the ones that integrate to 1) to define R, G, and B, and thereby get a more or less normal neutral for the colorspace, at E. Now, I still need to find a book that will verify that I've got this right... Here's a clue. Dicklyon 01:13, 8 April 2007 (UTC)
No they are not. The weights change when different primaries are used. They go with the normalized color matching functions, not the power spectrum. The weights do not change when the spectrum changes. Their function is to unnormalize the normalized color matching functions so that they can actually be used. For each set of primaries there are 2 sets of weights so that you can integrate either with a power spectrum (unnormalized to the human eye) or with a luminance spectrum (normalized to the human eye). The spectral power distribution I(λ) is a quantity used in physics and has nothing to do with human vision. It is measured in Watts/Hz (unnormalized). (Radiant intensity in Watts/(m^2 Steradian Hz) is more appropriate here actually and this should be used instead.) The radiant power weights either have to be included explicitly in the integrals or a new radiant power color matching function has to be defined. Since the normalized color matching functions are explicitly defined on this page, and I(λ) is also strictly defined through the link to "spectral power density" the definitions for R, G and B as currently written are wrong. Bababoef 11 April 2007 (UTC)
- That doesn't seem to be in accord with the books I was checking. Do you have a reference? Dicklyon 00:44, 12 April 2007 (UTC)
If you start from xbar,ybar,zbar:
(x,y,y)=(xbar,ybar,zbar)n1
n1 normalization to 1
(R,G,B)=(x,y,z)*Mt-1
(r,g,b)=(R,G,B)n1
n1 normalisation to 1
(rbar,gbar,bbar)=(xbar,ybar,zbar)*Mt-1
(r,g,b)=(rbar,gbar,zbar)n1
(X,Y,Z)=(r,g,b)Mt
(x,y,z)=(X,Y,Z)n1
etc.........
Bercier (talk) 11:42, 6 February 2013 (UTC)
RGB
[edit]For WRIGHT (sheet 142) A re-determination of the trichromatic coefficients of the spectral colours.WRIGHT.
R,G,B design his primaries 650nm 530nm and 460nm
A color can be designed by:
so C=alpha.R(650nm)+beta.G(530nm)+gamma.B(460nm)
if you divide by alpha+beta+gamma
r1+g1+b1=1
C= r1.R+g1.G+b1.B
and you have only the chrominance (r1,g1,b1)
For (r1,g1,b1) see BROADBENT (sheet3)
http://www.cis.rit.edu/mcsl/research/1931.php
Bercier (talk) 13:50, 4 March 2013 (UTC)
Human gamut not a triangle
[edit]The statement bothers me somewhat. Its true, if you can't find 3 points in the gamut that contain the gamut, then its not a triangle, but its also not some other things too. In other words, the converse is not true - If you can find 3 points that contain the gamut, that doesn't mean it IS a triangle. Only when you introduce the other fact, that the gamut must be convex, does the converse become true as well. PAR 15:59, 16 April 2007 (UTC)
- True; I considered mentioning convexity after I realized my statement was incomplete. Feel free to remove or fix it. Dicklyon 17:06, 16 April 2007 (UTC)
Creating the horseshoe gamut
[edit]I'm trying to understand how the CIE 1931 chromacity diagram is created and mapped to the horseshoe gamut. I experimented in MATLAB and wrote the following code with the output image at right. The output looks somewhat different from Image:CIExy1931.png (for example, the luminosity bands in Image:CIExy1931.png from the 460nm point on the border to 550nm and from 470nm to 605nm are not present in my image).
%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%% % CIE 1931 color space %%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%% A = [256:256:3]; for i = 1:256, for j = 1:i, A( i, j, 1 ) = ( ( j - 1 ) / 256 ) * 255; end; end; for i = 1:256, for j = 1:i, A( i, j, 2 ) = ( ( 256 - i ) / 256 ) * 255; end; end; for i = 1:256, for j = 1:i, A( i, j, 3 ) = 255 * ( 1 - ( ( 256 - i + j ) / 256 ) ); end; end; for i = 1:256, for j = 1:i, rgb_max = max( A( i, j, : ) ); A( i, j, 1 ) = ( A( i, j, 1 ) / rgb_max ) * 255; A( i, j, 2 ) = ( A( i, j, 2 ) / rgb_max ) * 255; A( i, j, 3 ) = ( A( i, j, 3 ) / rgb_max ) * 255; end; end; A = uint8( A ); figure, imshow( A );
Mainly I'm curious about how an image such as mine is mapped to the horseshoe gamut. Perhaps this information should be in the article?
Are the regions outside the horseshoe simply clipped, or is there a geometric transformation which occurs? There is far more pure red, green and blue in my image than in the official CIE 1931 chromacity diagram. Thanks! ~MDD4696 23:04, 20 April 2007 (UTC)
- Interesting, my image looks very similar to the first "rogue" image at http://www.techmind.org/colour/rogues.html. ~MDD4696 23:08, 20 April 2007 (UTC)
It's really not clear what you're doing here; seems to be a pure RGB hack with no reference to XYZ. You need to start with the data tables for the X, Y, and Z curves, parameterized by wavelength to get the horseshow. You can then compute for each x,y in the image what a good (X,Y,Z) is, and transform it by matrix to an sRGB image, and show that; clip when outside the horseshoe if you only want to show color corresponding to physical (non-negative) spectra. Dicklyon 23:10, 20 April 2007 (UTC)
- So, if I interpret you correctly, I'd need a table of wavelengths which specifies values for X, Y, and Z (red, green, and blue) for each wavelength to create the horseshoe. I'm confused about what you mean by choosing a good X,Y,Z from the normalized x,y values though. Thanks for responding. ~MDD4696 23:31, 20 April 2007 (UTC)
- The simplest thing is to choose X=x, Y=y, and Z=1-x-y. But you might want to scale them differently; or convert to RGB and then scale the largest to 255. Find CIE XYZ data through the links at the top of this page. Dicklyon 00:03, 21 April 2007 (UTC)
- Click on the chromaticity diagram to get information about the image. It has a description of how it was generated. The r,g,b numbers that you use are assumed to define a particular color or chromaticity in the sRGB color space. There is then a transformation (described in that article) on how chromaticities in sRGB can be converted to CIE XYZ coordinates. The sRGB gamut is smaller than the CIE XYZ gamut, and when the color in the CIE diagram steps outside the sRGB gamut, the color is set to a presumably "close" value. (I think if you draw a line from the D65 white point, the color on that line outside the gamut will be a constant, equal to the color where it first steps outside the gamut). Also - I *DO* see a white star in your diagram! I don't know why you dont. PAR 06:30, 21 April 2007 (UTC)
- There is a white star in mine, but there are also a few "bands" not present in mine in addition to the star. Thanks for trying to explain this all to me, I think I'm slowly figuring it out. ~MDD4696 02:35, 22 April 2007 (UTC)
One issue that might be missing here is that CIE is dealing with linear transformations while the display is extremely non-linear by design. That's why colors look wrong with problems like stars and such. First, the RGB values must be appropriate for the primary colors used by most computer monitors. So here I will call it Rm, Gm, and Bm, with m standing for monitor. In Windows, right-click once on the desktop and select properties --> Desktop tab, Color dropdown, then the Other button to get the Color window. Transformation between Hue Sat Lum over to R G B is completely wrong. This tells me that in Windows, the values you need to enter in a program are Rm' Gm' Bm', not Rm Gm Bm. So once you obtain the Rm Gm Bm values for the power to be emitted by the screen pixels, you then must apply gamma to obtain Rm' Gm' Bm' that you enter into your program. An approximation using a simple power law follows.
Rm' = Rm ^ 0.37 , Gm' = Gm ^ 0.37 , Bm' = Bm ^ 0.37
It's the Rm' Gm' and Bm' values that you enter into your program. This compensates for the extreme non-linearity intentionally designed into the monitor. Next you should put a dotted triangle to show the area that displays correct colors. Outside the triangle is artistically determined to make the diagram look pretty. Ghidoekjf (talk) 09:11, 27 June 2010 (UTC)
How is Y parameter a measure of brightness?
[edit]Our article says "the Y parameter was a measure of the brightness". It's not only an inexact starting point for our math, it also doesn't fit to the rest of the article. Since y is (apart from normalization) the same as Y, how come the diagram shows no increase in brightness from bottom to top? The cyan and the white at y=.3 look brighter to me than the green at y=.8. What's wrong: That statement, the definition of y, or the picture? — Sebastian 18:44, 8 June 2007 (UTC)
- The correct term is luminance (relative). Y is luminance, but y is normalized so that it's a pure chromaticity coordinate; if you double the intensity (brightness, power, luminance) of a color, Y doubles and y stays unchanged. In the xy space, luminance is unspecified, so it can be rendered with whatever luminance pattern you like. I don't think there's much wrong, but maybe the more precise word would be better. Dicklyon 02:21, 9 June 2007 (UTC)
Thanks for your reply, but it still doesn't make sense to me. I assume, by "xy space", you mean the projection of xyz space onto the xy plane (by neglecting z). If that's what you mean then it is not y Y = luminance that's unspecified, but z. Or did you mean a projection on the xz plane? — Sebastian 22:33, 9 June 2007 (UTC)
- Yes, xy is the projection of xyz, ignoring z. Since xyz is by definition normalized to x+y+z = 1 by dividing X, Y, and Z by X+Y+Z, y is not proportional to Y. Dicklyon 23:06, 9 June 2007 (UTC)
I see! I was confused by the word "normalization" which for me suggested a normalizing constant, but of course 1/(X+Y+Z) is not a constant. Are you sure "normalized" in the article is the right term? — Sebastian 00:32, 10 June 2007 (UTC)
- To me, normalized means adjusted by a factor to make it a predetermined size, in this case as measured by an L1 norm (Manhattan distance). Dicklyon 01:01, 10 June 2007 (UTC)
Funny, that similarity between "norm" and "normalization" never occurred to me! I'd say, it's coincidential, though. Is "normalization" really commonly used in the context of the color space, or is that just our article? In the first case, I'd vote for an explanation so others don't run in the same misunderstanding as I did. When I read "normalization", I always think: "It's just a formality, not a new physical aspect." — Sebastian 02:24, 10 June 2007 (UTC)
- I too do not understand the sentence about Y is the measure of intensity. First of all at that point of the article, and in the math formulas below it is not been told what X, Y, Z are. It is not even told what x and y are, but if X, Y,, and Z were defined, than the formulas would be enough to define them. I can suppose, but this is jus my opinion, that is common, to specify numbers as (x,y,Y) and you are referring to that in the sentence
- The derived color space specified by x, y, and Y is known as the CIE xyY color space and is widely used to specify colors in practice.
- However here Y is not the same Y that appears in the math formulas. This is just confusing. And this confusion is reflected in the article. -- AnyFile 11:59, 21 August 2007 (UTC)
- I too do not understand the sentence about Y is the measure of intensity. First of all at that point of the article, and in the math formulas below it is not been told what X, Y, Z are. It is not even told what x and y are, but if X, Y,, and Z were defined, than the formulas would be enough to define them. I can suppose, but this is jus my opinion, that is common, to specify numbers as (x,y,Y) and you are referring to that in the sentence
What is Y?
[edit]The introduction says "X, Y, and Z [are] roughly red, green and blue, respectively". But section "The CIE xy chromaticity diagram" says: "the Y parameter was a measure of the brightness or luminance of a color". Which one is it? The two are certainly not the same as can be seen by the fact that yellow (586 nm) appears brighter than green (546 nm). — Sebastian 00:32, 10 June 2007 (UTC)
- It's both. It's exactly luminance, by definition, and it's highly correlated with, or "roughly", green. Dicklyon 00:58, 10 June 2007 (UTC)
- Thank you. How are the other two, X and Z, defined, then? If one can see them as weighing functions, do they have negative values at blue resp. red? (Maybe the answer is somewhere in our articles or maybe I could ask this in a better way; I'd have to study this some more.) — Sebastian 02:30, 10 June 2007 (UTC)
- They were picked to be a non-negative basis for the 3D subspace of spectral space spanned by the human cone sensitivities. So, no, they are not negative anywhere, though most other sets of color-matching functions are. The Z is picked to be the narrowest possible non-negative function on the blue end, and then X is left as the smallest possible non-negative final dimension, which ends up with a bump on the blue end as well. Dicklyon 05:34, 10 June 2007 (UTC)
- Thank you. How are the other two, X and Z, defined, then? If one can see them as weighing functions, do they have negative values at blue resp. red? (Maybe the answer is somewhere in our articles or maybe I could ask this in a better way; I'd have to study this some more.) — Sebastian 02:30, 10 June 2007 (UTC)
- Can the article be updated to reflect this? I believe it is good practice to define terms before using them. I do not understande this topic enough to do that myself - that was why I was reading it! 81.178.102.250 15:34, 10 July 2007 (UTC)
- Good idea. I'll see if I can find a ref... Dicklyon 15:41, 10 July 2007 (UTC)
- At constant radiance, yellow is not brighter than green. It looks that way on a computer because "pure yellow" is red+green, which will be brighter than just green. If we normalised the xy diagram to be even vaguely constant-intensity in sRGB, most of it would look pretty dark — the xy diagram is only supposed to approximate chromaticity. ⇌Elektron 02:26, 30 August 2007 (UTC)
- Y, or luminance, was just an incorrect term. Because the human eye is so much more responsive to greens (look at the charts), the Y often correlates to brightness. Brightness should actually be X+Y+Z, or intensity. Y should not be used, and Intensity should be. In other words, Y is "perceived luminance" —Preceding unsigned comment added by 70.42.127.107 (talk) 11:07, 7 June 2010 (UTC)
- There are two concepts here, the luminance of a light beam and the intensity of a light beam. The luminance of a color is a measure of how "bright" it appears to the human eye, the intensity is a measure of the total physical energy being delivered in a light beam, a concept which does not involve the human eye. The Y chromaticity value gives the luminance of any color. Y does not "often correlate" to brightness, it is the scientific definition of brightness, as measured by experiment. It is the integral of the spectral power density times the function over all wavelengths . The intensity is simply the integral of over all wavelengths. X+Y+Z is NOT intensity, because does not equal unity at all wavelengths. For example, the X, Y, and Z values for a beam of light in the microwave region are all zero, yet the intensity is not. The functions are not the only possible functions that could be used. Any set of functions that covered the visible spectrum could have been used. The developers of the CIE functions decided to make the function serve double duty, in order to save on computation. It would not only represent a particular band in the greenish area of the visible spectrum, but would also give the luminance of the color being evaluated. PAR (talk) 15:38, 7 June 2010 (UTC)
Sorry, but I can not see it
[edit]It is written in the article, in the point list describing the diagram
- It is seen that all visible chromaticities correspond to non-negative values of x, y, and z (and therefore to non-negative values of X, Y, and Z).
Sorry, but I really can not understand the presence of this sentence. That all visible color could be written by saying 3 positive numbers X, Y, Z was an hypothesis, so you can not conclude that. Moreover it has not been proved that all the color are in the diagram (by the way I can not see brown there) -- AnyFile 12:25, 21 August 2007 (UTC)
Moreover I can not understand why the border of the gamut are where they are in the figure. Why, for example, the point (x=0.1,y=0.85) is outside the border? Of course I can understand that it could not be that x+y>1 (because that was how x and y was defined), but not why for example the couple I wrote above can not be in the visible part.
Is it that if a light beam of components that lay outside the gamut is seen by an human eye, it is not seen by the human eye? (I am strongly feeling as all of this description is not about the colors, but about the perception of colors by human eyes).
And why is not the point (1,0) inside the gamot? If a pure red is X=1,Y=0,Z=0 than it should be a x=0,y=0 (and the averall intensity Y=1). -- AnyFile 12:57, 21 August 2007 (UTC)
- That all colors can be represented by 3 numbers X,Y, and Z is an experimental fact, it is found by experimentation. It cannot be "proven" in a mathematical sense, and it is not a "hypothesis". If X,Y, and Z actually do represent every color, then it follows that the chromaticities x and y will represent every chromaticity.
- The color brown is simply the color yellow at low luminosity. Brown and yellow have the same chromaticity.
- With regard to the (0.1, 0.85) point - The first thing you have to understand that the curved portion of the gamut is the line of monochromatic colors. This is an experimental fact. These points correspond to light at a single wavelength. Every light beam can be thought of as a mixture of a number of different monochromatic beams. The second thing you have to understand is that the human eye is linear (Grassmann's law). This too is an experimental fact. This means that for two points on the xy chromaticity diagram, any mixture of the color corresponding to these two points will lie on a line connecting the two points, and will lie between them on this line. It also means that any color can be constructed as the mixture of two monochromatic colors. The (0.1, 0.85) point is not on the monochromatic line, therefore it must be a mixture of monochromatic colors. You cannot find any set of monochromatic colors that will mix to give (0.1,0.85). Geometrically, you cannot find two points on the monochromatic curve such that (0.1,0.85) lies between them! This is an experimental fact that is derivable from the two experimental facts mentioned above.
- You say this article "is not about the colors, but about the perception of colors by human eyes". This is a bad statement. There is no such thing as "color" that is independent of the eye. Without human eyes, there is no color, there is only light with different spectra.
- With regard to the (1,0,0) point, pure red is NOT (1,0,0) in the XYZ space. It is pure red in the sRGB space. We are not talking about sRGB here.
Converting a full set of chromaticities (xy for RGB+W) to a full set of triplets (Yxy/XYZ)
[edit]The sets of RGB-primaries along with white point are often defined with chromaticities solely, i. e. 4 xy-pairs for red, green, blue and white. For example, this is the only information stored in EDID, as well as numerous reference tables of standard RGB spaces throughout the Wikipedia and many (but not all) other sources.
How these chromaticity sets can be converted to XYZ- or Yxy-sets?
Of course, within common mathematics we can't just add 4 single values for luminance — there will always be some 4 degrees of freedom. But with the concept of that R+G+B=W and the assumption that we don't need absolute Y-values, just relative ones (i. e. fixing luminance of white point to Y=1 or Y=100), it sounds possible.
As a result, I developed some equations, starting with Xr+Xg+Xb=Xw, etc.
- The source chromaticity data: .
- Additionally, we fix (or any other positive value, maybe 100).
- Some intermediate variables (as in Yxy->XYZ, but without Y-multiplier):
- Some auxiliary variables (differ only in first X'; the subscript G is referring to the RGB-primary we start with):
- After some reductions, we finally have the answer:
As you can see, all this seems to give unambiguous results. But, despite of looking very credible and passing all the checks (by definitions), the results for well-known RGB spaces, such as sRGB or Adobe RGB, don't match predefined values of Y (refer to Bruce Lindbloom). Could someone tell me what I'm missing? Which degree of freedom was not eliminated, and how could this happen with such precise formulae?
For your convenience, I've prepared a small Excel spreadsheet which does these calculations automatically. It can be downloaded here: avsco.nm.ru/EDID2XYZ_eng.xls (only 17 Kbytes in size, no archiving).
Any hints are appreciated. Thanks in advance. 213.234.235.82 11:26, 17 October 2007 (UTC)
- I haven't studied it in detail yet, but where you say R+G+B=W and Xr+Xg+Xb=Xw, you probably mean to use R=G=B at whitepoint or something like that. That is, spaces are specified such that equal amounts of the primaries add to form the color defined as white. Dicklyon 17:30, 17 October 2007 (UTC)
- This topic is about primaries, i. e. red, green, blue and white at maximum intensity. 213.234.235.82 17:42, 17 October 2007 (UTC)
- Primaries and whitepoints are chromaticities, independent of intensities. Dicklyon 04:17, 18 October 2007 (UTC)
- Color stimulus is unthinkable without intensity. My question is about primary stimuli of a real display device, which has certain luminocity aside from chromaticity. Only this way the sum of red, green and blue can render the specified white point (no matter whether Y is normalized to 1 for white or not). Otherwise, if we just take some arbitrary "reddish", "greenish", "bluish" and "neutral" stimuli, which do not form a single system, the conversion from 4×xy to 4×XYZ would not be possible, of course. 62.118.220.182 08:40, 18 October 2007 (UTC)
- But if you're going to asking for help, listen to the answers (and preferably not on an article talk page, since this is supposed to be about article content; but I'll assume that's where you're trying to go with it). A chromaticity is a color with intensity factored out. An xy specification of the primaries doesn't tell you how intense those primaries are, and in general they are variable, the max limit notwithstanding. The whitepoint is a specification of the neutral chromaticity that you get by producing "equal" amounts of the three primaries; a color at the whitepoint chromaticity can be white, gray, or even nearly black. By "equal" is meant that the colorspace specifications of the primary intensities, generally called the R, G, and B values, are numerically equal; these multiply whatever the intensities of the primary sources are; for example, if you scale the R, G, and B range to be 0 to 1, then they multiply the maximum intensity of the primaries. If you just want to look at brightest white, that's R=G=B=1, but in general any neutral color has R=G=B and generates a color with chromaticity equal to the white point. There is no sense in which the sum R+G+B is a meaningful quantity in colorimetry as I know it. Hope this helps. Dicklyon 14:54, 18 October 2007 (UTC)
- As for help figuring out why your specific formulas are giving you wrong results, you might try emailing Bruce Lindbloom directly. He has in the past been quite responsive and helpful. Or perhaps for a broader response, try the Apple ColorSync users mailing list. --jacobolus (t) 20:14, 18 October 2007 (UTC)
Red, Green and Blue Not Objective Concepts,
[edit]The article manages to imply that there are objective color values that are universally recognized as red, green, and blue. It should be more clear about the fact that the primary colors used in any color model are chosen to make the model work, not derived from objective fact. --Isaac R 17:58, 18 October 2007 (UTC)
Isn't the word tristimulus counterintuitive?
[edit]
From what I understand, the XYZ- or xyz-space has been chosen so that the so-called "desirable properties" would be met. For instance, the Y value had to be equivalent to the experimental luminosity of the different wavelength to the human eye. And the X and Z values were defined in a purely mathematical way so as to fit the gamut as best as possible, and so that the other conditions would be met, such as being positive everywhere, etc. For instance, if I was to take the values on the graph here, normalise them so that the sum is 1 for each wavelength, I would get a parametrisation of the horsehoe shape on the chromacity diagram.
Now, on the Tristimulus page, it is said that tristimulus values are "the amounts of the three primary colors in a three-component color model needed to match that test color. The tristimulus values are most often given in the CIE 1931 color space, in which they are denoted X, Y, and Z."
Something is wrong. From the definition, tristimulus values is the amount of the three primary colors needed to match a test color, (sometimes "negative", unless the gamut is a perfect triangle, which it is not). In this case, there is a color corresponding to each positive set of values, since they correspond to the superposition of (existing) primary colors. However, in the case of X,Y,Z, there are triples not corresponding to any color, such as (1,0,0), (0,1,0) and (0,0,1). X,Y,Z are not supposed to correspond to any primary colors. Or do they correspond to convenient "imaginary" colors that are of no particular interest, except for generating a nice parametrisation? If this is the case, I'll add something to that effect on the Tristimulus page. Ratfox 23:29, 31 October 2007 (UTC)
Is there any tables to generate the images in the article?
[edit]What research is done that results the images in the article (the curves)? There must have been some research project behind the images with the curves in, from which the images then is generated. Is it possible to get the result of that research, for further researching? Or at least the data the images is generated from?
Since the images could be analyzed and data extracted from the curves, the data should be publicated somewhere to get. An analyze would be such a work around... :-\
-Kri 23:25, 11 November 2007 (UTC)
What are the extremes of the visible colour spectrum?
[edit]What I mean is, what range of wavelengths are considered to be part of the visible colour spectrum, as opposed to infrared or ultra-violet? I can see from the article that it's roughly 400-700 nm, but I wondered whether there is a more precise definition used by the CIE, or other standards body. For example, the article visible spectrum uses the range 380-750 nm. Do those values come from the CIE? (a reference would be appreciated). Thanks, Thunderbird2 (talk) 12:03, 12 January 2008 (UTC)
Oh, and defining wavelength ranges for individual colours would be nice too :-) Thunderbird2 (talk) 12:11, 12 January 2008 (UTC)
- There are a number of different tabulations of the CIE color matching functions, beginning with the 1931 standard version which covered 360-830 nm. The "abridged" values were a more "user friendly" version (back when calculations were done by hand) and they ran from 380-780 nm. There are others, 1964 improved version, etc. etc. I don't know if there is a set of numbers that the recent literature has settled on, but the 360-830 probably covers everything. Check out Wyszecki and Stiles ("Color Science") for tables of about twenty different versions of color matching functions. PAR (talk) 17:10, 12 January 2008 (UTC)
- But with respect to the original question, parts of that extended range would certainly be considered IR and UV as opposed to visible, even though it has some (very small) visible effect; 400 and 700 are the most common "conventional" boundaries, but I don't know that they have any special status. Dicklyon (talk) 18:12, 12 January 2008 (UTC)
- Thanks for your replies - both helpful. Thunderbird2 (talk) 18:44, 12 January 2008 (UTC)
I cannot understand how anything shorter than about 395 nm ever got included in the visible spectrum in the first place; as to repeated instances, it appears that some sources just copy from others without bothering to check. In my own experience, even at 395 nm the color I saw from the source was not violet; in addition, light at 395 nm excites significant fluorescence in the lens of the eye. By 390 nm the fluorescence (and perhaps other effects) dominated so thoroughly that I got no impression of violet at all, and in fact no sense of light from the source, just an overall nondirectional blur. It makes much more sense to me to use 400 nm as the short-wavelength end of the visible. As to the long-wavelength end, I like the idea that by about 700, small changes in wavelength produce minimal changes in perceived color. That said, it seems clear that the long-wavelength end of visibility is extremely variable; for convenience I generally cite the visible spectrum as a full octave, from 400 to 800 nm. Jonsinger (talk) 21:39, 13 November 2015 (UTC)
CIE Standard colorimetric observer
[edit]Please can someone write a separate section—or even article—to explain what it is and why it is needed. I'm not comfortable with the flow of the article (to put it mildly) so I don't know where to insert it myself.--Adoniscik (talk) 01:36, 21 January 2008 (UTC)
- It should not be a separate article. The standard observer is the set of three spectral sensitivity curves that are used to convert a spectrum to an XYZ tristimulus vector; other observers (set of curves) will give other kinds of tristimulus values. Dicklyon (talk) 02:23, 21 January 2008 (UTC)
- The reason you might make it an article instead of a section is that the 1931 definition isn't the only one. In any case, it needs more attention so people flocking from other articles where the concept is mentioned do not have to read the entire article to get a definition. It's scattered right now.--Adoniscik (talk) 02:33, 21 January 2008 (UTC)
- I made a section up front where it will get noticed. I'm generally against article proliferation. Dicklyon (talk) 02:48, 21 January 2008 (UTC)
- They're going to have to read some of this article anyway to understand the standard colorimetric observer; too much necessary context overlaps between this article and that one. I also oppose article proliferation in this case, unless some section of this article becomes so long and detailed that it must be split out. --jacobolus (t)
Undo removal of external links
[edit]Why?
- The so called (→External links: clean up) removed the links I typically use on this page
- But dead links were NOT removed
- This is exactly what a link clean-up NOT should do
http://cvrl.ioo.ucl.ac.uk/ —Preceding unsigned comment added by 80.156.4.26 (talk) 19:24, 27 February 2008 (UTC)
Primary???
[edit]It seems to me that the word "primary" is bandied about as though it means something special -- whereas in most cases in this and other Wikipedia articles on color, it means no more or less than the word "color".
In those cases, the word "color" would be far clearer to the reader.Daqu (talk) 14:27, 9 September 2008 (UTC)
- I've searched the article on the word "primary" and in every case it is used appropriately. The word "primary" DOES mean something special. The CIE space is based on three specific, fixed colors, called "primary colors". All other colors are defined as mixtures of these three primary colors. "Primary color" should never be confused with the generic term "color". PAR (talk) 16:25, 9 September 2008 (UTC)
- It's perhaps more subtle than that. "Primary" is a usage of a color; any color can be used as a primary. And primaries are often not real colors; the XYZ primaries are "imaginary" in the sense that they do not correpond to any real color, that is, to any color that corresponds to a non-negative light spectrum. Dicklyon (talk) 18:18, 9 September 2008 (UTC)
- Absolutamundo. I was restricting myself to the CIE case, because I thought anyone who thinks "primary color" means "color" is probably not ready for the deeper explanation. PAR (talk) 18:13, 10 September 2008 (UTC)
- Well, I am familiar with the 1931 CIE diagram, the color matching experiments that led to it, and how to simulate a chosen triangle in the CIE diagram with computer graphics using RGB space. But I can also read, and it is clear that even if "the word primary is being used appropriately", it is not being explained to the reader. Does it merely mean the vertices of a chosen triangle in CIE color space? Whatever the answer, it is not made clear in this article.
- Oh, and PAR, you can lose the condescenscion.Daqu (talk) 15:49, 12 September 2008 (UTC)
- I meant no disrespect to you, I was explaining myself to DickLyon. There was a time when I was not ready for a deeper explanation too. In fact, I agree with you that the meaning of the word primary should be clearer in this article. Don't be so paraniod. PAR (talk) 18:10, 12 September 2008 (UTC)
The phrase 'Primary Color' is unfortunate. In school we are told that with Red, Green and Blue lights we can make any color and so these are Primary Colors. Of course this is rubbish because you cannot generate all colors by mixing any single set of three colors. Consequently there are no real primary colors. Alternatively you can call any three non-colinear colors restricted Primaries. Whilst this is simple, many people are hung up on the concepts they were taught in school. My personal choice would be to eschew the phrase 'Primary Color' altogether.OrewaTel (talk) 01:45, 11 June 2019 (UTC)
Significant digits in Matrix
[edit]"The numbers below all have the correct number of significant digits per CIE standards." Does this mean that all of the digits defined by the CIE standards are given (and thus all source information is kept) or that the numbers have been rounded (and thus source information is lost) according to error limits given in the standards? Please disambiguate this sentence!--SiriusB (talk) 21:29, 13 April 2009 (UTC)
How come the matrices don't show up when the article is saved as a PDF? -- 85.64.97.123 (talk) 21:49, 1 November 2010 (UTC)
RGB color matching function
[edit]
How exactly has this image been created? The description page only says that the data have been retrieved from here, but on that page there is no RGB CMF, just XYZ CMFs. Has the inverse of the RGB-to-XYZ conversion marix been used? If so, please add this info to the description page. On the cvrl site are other RGB CMFs that differ greatly from this one. In particular, the red curve of the Stiles & Burch (1955) (text file) CMF peaks near 3 while blue and green are both around 1.--SiriusB (talk) 21:49, 13 April 2009 (UTC)
Revert to previous image of color space
[edit]I have reverted the replacement of the CIE 1931 Chromaticity Diagram for the following reasons:
- This was not a fixed picture, it is a damaged picture. Removing the "sRGB line artifacts" destroys the color accuracy inside the sRGB triangle. In the original, these colors were carefully reproduced from the xyz values of the sRGB colors, and the "line artifacts" are the unavoidable result of this accuracy.
- The description of the original (CIExy1931.png) gives an exact explanation of how the original color diagram was generated. If the image is replaced, the description should be altered to reflect the exact method by which the new image was generated. This .png image was then replace by a somewhat inferior .svg file (CIExy1931.svg) which nevertheless reproduced the colors accurately. I have copied the description of how the colors were generated over to the description of the .svg file.
- sRGB cannot accurately represent colors outside the sRGB triangle, so the statement that the colors outside the sRGB triangle are "more accurately represented" is questionable at best, and wrong at worst. The method by which the colors outside the triangle are generated were stated in the original. The same reasons should be explicitly stated for any replacement and the reasons why they are more accurate than the original should be given.
PAR (talk) 23:42, 11 June 2009 (UTC)
- BenRG has now remade the new svg now, with good explanations of exactly what he did. I agree with him that eliminating the various line artifacts is more important than trying to minimize the pointwise error inside the sRGB gamut region, as it makes the image a visually better representation of what it's trying to represent, within the gamut limitations of sRGB. Dicklyon (talk) 03:13, 25 September 2009 (UTC)
- The numbers on the left cause the entire work to be rather unbalanced. In fact, I find that all the numbers are visually rather annoying, conveying an aura of materialistic obsession. Also, the sharp red corner on the right is really at odds with rest of the diagram and should be rounded a bit, don't you think?. There are still rather sharp gradations of color, but these are acceptable, indeed desirable, since they are indicative of the artist's existential struggle with his inner self within the context of the multifaceted aspect of cosmic reality. :) PAR (talk) 09:10, 25 September 2009 (UTC)
Irritated by the 2nd paragraph
[edit]Is it just me or is
"The CIE XYZ color space was derived from a series of experiments done in the late 1920s by W. David Wright[3] and John Guild.[4] Their experimental results were combined into the specification of the CIE RGB color space, from which the CIE XYZ color space was derived."
really saying CIE XYZ -> CIE RGB -> CIE XYZ ? —Preceding unsigned comment added by 93.133.208.217 (talk) 17:33, 23 August 2009 (UTC)
- Looks to me like it says measurements -> CIE RGB -> CIE XYZ, which is about right. Dicklyon (talk) 03:14, 25 September 2009 (UTC)
"White"
[edit]I have noticed that this has been brought up before, but the current wording in the article is (presumably) as confusing now as it was when this was discussed before. Section CIE_XYZ#The_CIE_xy_chromaticity_diagram_and_the_CIE_xyY_color_space reads "Less saturated colors appear in the interior of the figure with white at the center." I understand the text is meant to talk about white in a "zero-chromatic", "white point" sense – but it doesn't do the job properly. Especially since the word "white" is actually linked to the article white, which obviously talks about white-white, not "white point" white. Given the number of purists around here I was reluctant to be bold, since I was afraid I'd risk ending up with something even worse – but please, cut regular folk some slack and reword that for clarity!
Having said that, is there a given top level in the xyY shape of the human gamut, at least in theory? (In practice there must be a limit, since your retina would surely burn if you pushed the luminance too high, but I haven't found any definitive answer regarding the theory.) I assume that if there is one, the top level should be a point, just like the section at Y=0, since there's no possible chromatic variation with pure white -- is that correct? Speaking of which, is there any representation of that 3D shape in its entirety? I understand it would serve little practical purpose, especially since from the outside you'd see mostly colors outside the gamut of any device, but I think it would be a good helper for putting the concept into perspective, if you'll excuse the pun. Oh, and finally, in such a three-dimensional representation, what would be the shape of the section we're used to see? Would it be a plane parallel to xy? A spherical cap? A paraboloid? None of the above? --Gutza T T+ 02:44, 27 September 2009 (UTC)
- It looks to me like White describes the concept as described in this article. White, in terms of chromaticity, just means neutral; there's no intensity implied, and there's not max white in xyY or XYZ space. There is no bounding 3D shape for these spaces. A chromaticity diagram is a slice through the space, anywhere that cuts in with a finite cross section, and not including the origin; xy and uv and rg are examples; they all look fairly similar, just stretched differently. Dicklyon (talk) 03:09, 27 September 2009 (UTC)
Thank you for the prompt answers -- you're right, White is consistent with the concept described here. Also, upon further consideration it makes sense that the shape is not bound towards +Y, since you can get any close you want to the Sun (probably burning your retina in the progress) and you still won't think it's white. Wouldn't it make sense to include the gist of this in the article, though? --Gutza T T+ 03:34, 27 September 2009 (UTC)
- It depends on what you’re interested in. For colors of surfaces, often a perfectly diffusing reflector is taken to be “white”. For light sources, there’s no upper limit to luminance, but when the eye has adapted to some particular surroundings, there is some brightness beyond which the eye’s photodetectors will no longer notice any differences. Take a look at http://www.scholarpedia.org/article/Color_spaces. –jacobolus (t) 22:30, 8 June 2010 (UTC)
Ok, that's gist allright. :) One last question though: is there any special reason they picked x and y among x, y and z? I mean, they could've picked any two of those, plus any one of X, Y and Z and the result would've been just as consistent. The choice for Y is obvious, but is there any reason for the other two specifically? --Gutza T T+ 04:23, 27 September 2009 (UTC)
- It's pretty arbitrary; I think they just picked the first two of the over-complete set of three. Dicklyon (talk) 17:29, 27 September 2009 (UTC)
- At the beginning, using x and z in a chromaticity diagram was also somewhat common. I believe (but am not positive) that the x–y diagram is from Judd. –jacobolus (t) 22:32, 8 June 2010 (UTC)
Thank you, that was my feeling as well, but I wasn't sure whether there was in fact a reason. I'll see if I can find a place to mention this in passing in the article. --Gutza T T+ 17:43, 27 September 2009 (UTC)
- There's no need for us to put our speculations or feelings into the article; find a source or don't mention it. Dicklyon (talk) 17:55, 27 September 2009 (UTC)
Fair enough – and I'm obviously unable to source that, or I wouldn't have asked in the first place. Oh well. --Gutza T T+ 18:09, 27 September 2009 (UTC)
Grassmann's law linearity is not due to visual system linearity
[edit]"One might ask: "Why is it possible that Wright and Guild's results can be summarized using different primaries and different intensities from those actually used?" One might also ask: "What about the case when the test colors being matched are not monochromatic?" The answer to both of these questions lies in the (near) linearity of human color perception. This linearity is expressed in Grassmann's law."
The linearity in Grassmann's law is due to the fact that it uses linear arithmetic to add amounts of light energy. By definition, the total light from two equal sources is twice that of one of the sources. In fact, human perception of light intensities is very non-linear, but this is irrelevant in color matching, since the two colors that match produce the same cone excitations. The relative visual response of unequal excitations is never compared in the determination of matches. Matching is the only situation to which Grassman's law applies, and it must apply by definition of the experimental conditions (matching) and the measurement units (intensity) involved.
Because of the linear arithmetic, other things also hold, such as the chromaticity of a mixture of two colors lying on a line connecting the chromaticities of the two components.
Old tv nut (talk) 01:26, 21 April 2011 (UTC)
- Doesn't the statement "(near) linearity of human color perception" rather than human perception of intensity make the statement correct? PAR (talk) 05:23, 21 April 2011 (UTC)
- It has to do with the (near) linearity of the independent cone responses with respect to different wavelengths. That is, if two different stimulus spectra produce the same color sensation, Grassman's law says that when you double the intensity of each stimulus, the two resulting stimuli will still produce the same color sensation (that is, the same as each-other; different obviously from the first one). It turns out that this statement is not quite empirically true. However, it is close enough to true to be useful in constructing models; without it, it is impossible to summarize the color sensation induced by some spectrum in terms of coordinates like XYZ or similar. –jacobolus (t) 05:37, 21 April 2011 (UTC)
The experiment described (two different stimulus spectra that match are each doubled in intensity and compared) results in an exact match between the two final doubled stimuli because both stimulate the cone cells equally. The linearity of the result occurs because the stimuli are measured on a linear scale of intensity. The fact that a match was obtained says nothing about the linearity of the visual system. In fact both doubled-intensity stimuli would appear to be about 25 percent brighter than the original stimuli (not 100 percent brighter), assuming the same surround conditions. A matching experiment is not affected by this non-linearity of response, and can give no indication of its existence or where it occurs in the visual system. It is very convenient that color matching can be described by linear arithmetic in the physical domain, but it does not come about due to any linear or even near-linear characteristic of the visual system. Old tv nut (talk) 19:32, 22 April 2011 (UTC)
- It's not linearity of the visual system as a whole, but it is linearity of the conversion from spectrum to effective stimulus. That is, the effective stimulus is a weighted intensity, weighted via the spectral sensitivity curves of each rod and cone type. Grassman's law essentially means that it's sensible to treat this as a dot product. Also, for it really to be true, the different cone types need to respond with similar nonlinearities or adapted gains at least, and the rods need to be out of the picture; so it doesn't work at very low (mesopic and scotopic) levels. Dicklyon (talk) 01:38, 23 April 2011 (UTC)
Modifications by Judd (1951) and Vos (1978)
[edit]The article does not mention any reference to the modifications of CIE 1931 by Judd (1951) and Vos (1978) [1]. And apparently, these modifications are essentially not used in any standard (including modern ones). Are these modifications officially rejected for some reason?--SiriusB (talk) 12:13, 5 September 2011 (UTC)
- I think you’d be best served by reading some basic color science or colorimetry book, instead of asking questions piecemeal across 5 different talk pages. These updated CMFs were never “rejected”, but they never gained much use, because there is no way to convert data collected assuming the CIE 2° standard observer to another set of CMFs, and so any official modification would make a break in measurement compatibility. Basically, the 1931 standard observer is “good enough” that maintaining compatibility between measurements is more important than the quite slight changes that would result from corrections. –jacobolus (t) 18:16, 5 September 2011 (UTC)
- If Wikipedia, including its discussion pages, requires the user to read all relevant literature first, before being able to understand the articles, rather than providing exactly this basic information (and the question why an 80 years old standard is still not yet replaced by an improved one is exactly such a basic and obvious one), then WP is doing a bad job and is rendering itself irrelevant. You are the second one replying to me in such a manner "read the books (which?) before consulting WP", and therefore the second argument to me to leave WP forever. The third may be the final one. WP has its best days passed. Really. BTW If you know any references to this "the 1931 standard is still good enough", why don't you just add this in the article?--SiriusB (talk) 10:30, 7 September 2011 (UTC)
- I’ll agree with you that Wikipedia’s articles on these topics are quite lacking in many ways. The problem is that it’s hard to synthesize any kind of coherent introductory material given that the organizational structure here demands articles strictly limited to one subject, and no one has really put much effort into writing clear and sufficiently complete basic pages that can be linked to from all the others. I’m not suggesting you find a book just to chase you away (indeed the articles here need all kinds of work if you’re willing to do the research and writing that it takes to improve them). I just actually think you’d get more out of time spent on a book than trying to learn only from Wikipedia pages. In re books, I think Hunt’s Reproduction of Colour is pretty good, or if you’re interested in CIE colorimetry more specifically, there’s a recent book called Colorimetry: Understanding the CIE System, edited by Schanda. Really though any book would probably be helpful, even ones that are 30 or 40 years old: any local public library probably has a couple. Why the CIE 1931 color space persists is actually quite a deep and tricky problem, and answering it properly would take a few thousand words. The reason I haven’t added everything I know about anything to Wikipedia is that time is quite a limited resource and the number of such things to be added is large. –jacobolus (t) 20:20, 7 September 2011 (UTC)
COLOR MATCHING FUNCTIONS
[edit]HOW TO FIND 0.49 0.31 0.20 0.17697 0.81240 0.01063 0 0.01 0.99?
sbar=xbar+ybar+zbar
x=xbar/sbar y=ybar/sbar z=zbar/sbar
(x,y,z)=(xbar,ybar,zbar)n1
n1 means normalization to unity
divide each term by the sum of the line.
V1924=ybar relative luminous efficiency.
X=.49r+.31g+.2b
Y=.17697r+.8124g+.01063b Y is the relative LUMINANCE.
Z=.01g+.99b
L=X+Y+Z=X/x=Y/y=Z/z
L=Y/y is the relative BARYCENTRIC LUMINANCE .
xbar=.49rbar+.31gbar+.2bbar
ybar=.17697rbar+.8124gbar+.01063bbar
zbar=.01rbar+.99bbar
we put D=d.r+e.g+f.b
V=V1924
rbar=r*V/D
gbar=g*V/D
bbar=b*V/D
so (rbar,gbar,bbar)=(r,g,b)*V/D who is one identity always right.
so S(A)=sum of 5 nm in 5 nm from 360nm to 830 nm of the function A
S(V1924)=S(xbar)=S(ybar)=S(zbar)=S(rbar)=S(gbar)=S(bbar)=S
By approximations on d,e and f you find:
for S(rbar)=S(gbar)=S(bbar)=S gives 0.17697 0.8124 and 0.01063
x>0 and z>0 gives by approximations 0.49 0.31 0.20 and 0 0.01 0.99
It's very easy with Excell.
- I have no idea what you’re asking about. –jacobolus (t) 20:50, 11 October 2012 (UTC)
mister jacobolus
What you don't understood?
Bercier (talk) 18:41, 13 November 2012 (UTC)
Bercier (talk) 18:37, 14 November 2012 (UTC)
Bercier (talk) 11:17, 4 March 2013 (UTC)
Bercier (talk) 13:18, 29 May 2013 (UTC)
X,Y,Z comments moved here from article
[edit]I removed this from the end of the article, and copied it here with minor formatting chagnes. Dicklyon (talk) 16:22, 12 November 2012 (UTC)
Meaning of X,Y and Z
[edit]In this model, Y means luminance, Z is quasi-equal to blue stimulation, or the S cone response, and X is a mix (a linear combination) of cone response curves chosen to be nonnegative. Thus, XYZ may be confused with LMS cone responses. But in the CIE XYZ color space, the tristimulus values are not the L, M, and S responses of the human eye, even if X and Z are roughly red and blue. Rather, they may be thought of as 'derived' parameters from the long-, medium-, and short-wavelength cones.
The CIE has defined a set of three color-matching functions, called , , and , which can be thought of as the spectral sensitivity curves of three linear light detectors that yield the CIE XYZ tristimulus values X, Y, and Z. The tabulated numerical values of these functions are known collectively as the CIE standard observer.
- is a 3x3 matrix
- is a 3x3 diagonal matrix diagonal terms are 330 286 55.
For CIE1931 2° xyY
LUMINOUS EFFICIENCY
EQUIENERGETIC LIGHT
- is the relative LUMINANCE.
- is the barycentric RELATIVE LUMINANCE :
For having :, you have to integrate for dλ ->0 like for a ray of light(monochromatic light).
THEN:
then:
If:
x=Xa/(Xa+Ya+Za)=xbar/(xbar+ybar+zbar)
y=Ya/(Xa+Ya+Za)=ybar/(xbar+ybar+zbar)
z=Za/(Xa+Ya+Za)=zbar/(xbar+ybar+zbar)
If:
we can write more simply:
so:
n1 normalization to unity
divise x,y,z by the sum x+y+z
Bercier (talk) 10:45, 10 April 2013 (UTC)
Bercier (talk) 13:34, 29 May 2013 (UTC)
Discussion
[edit]I removed something like that from the article once before. It's not clear what the "X a" notation is supposed to represent, or why the non-relevant term "LUMINANCES" is introduced here, or whether there's an important point being made in the change of the limits of integration, or where the coefficients come from in the rgb matrixing (CIE RGB? sRGB? LMS? or what?). WIth some clarification, including links to a relevant source or two, maybe we can see what problem Bercier is trying to solve. Dicklyon (talk) 19:50, 12 November 2012 (UTC)
Ya integral is ABSOLUTE LUMINANCE
[edit]Ya is one indefined integral but really one summation by 5 nm to 5nm between the extremities of the visible spectrum like tabulated by V1924=ybar by the CIE 1931-2° also 360nm to 830nm.
For CIE xyY 1931-2° Ya is for calculation
1/if you take a ray of wawelenght λ
and for the equi-energetic spectre S(λ)=S
Ya=xbar*S
also y=Ya/(Xa+Ya+Za)
we have also y=Y/(X+Y+Z)
but Y( LUMINANCE ) is different of Ya:a for ABSOLUTE LUMINANCE
2/For all the spectrum from 360nm to 830nm
and for the equi-energetic spectre S(λ)
Ya is the area under the curves of the integral and the CIE 1931xyY-2° choose Xa=Ya=Za
3/ You have also:
Ra=Ga=Ba
r=Ra/(Ra+Ga+Ba)=g=b=1/3
4/To return to WRIGHT's(10) and GUILD's(7) experimentations
we have to inverse the matrix Mt
(rbar,gbar,bbar)=(xbar,ybar,zbar)*Mt-1
rbar=2,3635xbar -,5151ybar +,0052zbar
gbar=-,8958xbar +1,4264ybar -,0145zbar
bbar=-,4677xbar +,0887ybar +1,0093zbar
sbar=rbar+gbar+bbar
r=rbar/sbar
g=gbar/sbar
b=bbar/sbar
r,g,b are the calculed WRIGHT+GUILD'results with 2nm differencie with the WRIGHT+GUILD 's real experimentations(10+7) 1930.
5/we can re-start with r,g,b
Y est déterminé pour que les surfaces sous les courbes rbar,gbar et bbar soient égales entre elles et égales à la surface sous V1924. soit pour que Ra=Ga=Ba
soit D=dr+eg+fb qui est une identité
En calculant :
(rbar,gbar,bbar)=(r,g,b)*V1924/D on trouve par ajustements successifs
equivalents à la méthode des moindres carrés ou à la méthode d'approximations successives de NEWTON ou à un calcul itératif:
donc on trouve
d=0.17697 e= 0.8124 f=0.01063
On détermine ainsi Y=0.17697r+0.8124g+0.01063b la LUMINANCE à un coefficient 1/0.17697=5.65(08) près qui ne joue pas sur les différents calculs.
Ensuite en écrivant que xbar et zbar(donc x et z) ne comportent aucune valeur négative et que le diagramme y(x) est tangent aux axes on trouve par ajustements successifs
en calculant xbar=arbar+bgbar+cbbar et zbar=grbar+hgbar+ibbar:
a=0.49 b=0.31 c=0.2
g=0 h=0.01 i=0.99
ce qui permet de calculer
X=0.49r+0.31g+0.2b et
Z=0.01g+0.99b
et donc la luminance relative barycentrique L=X+Y+Z qui permet avec le gamut de déterminer le mélange de n lumières comme barycentre des points x,y,z affectés du poids de leur luminance relative Ln.
all these calculations are very easy with Libre Office tableur
reference:BROADBENT
Bercier (talk) 12:33, 6 February 2013 (UTC)
Bercier (talk) 14:05, 13 November 2012 (UTC)
- Bercier, I don't follow. Maybe you can start by pointing out a source that distinguishes Ya from Y as you do. And we use the term "luminance" only for Y. It's not clear what term in English would be better for the "relative luminance Y/y" that you refer to; maybe tristimulus component Y? Dicklyon (talk) 15:34, 13 November 2012 (UTC)
I HOPE YOUR ANSWER THANK YOU
Bercier (talk) 19:09, 8 February 2013 (UTC)
Bercier (talk) 19:15, 1 March 2013 (UTC)
- Sorry, you link is not working for me and I don't follow your point. I don't see anything like the Xs notation. Are you just worrying about the imperfect precision of the sums of the CMFs? Dicklyon (talk) 22:54, 13 November 2012 (UTC)
http://www.cis.rit.edu/mcsl/research/broadbent/CIE1931_RGB.pdf
Calculation from the original experimental data of the CIE 1931 RGB standard observer spectral chromaticity Co-ordinates and color matching functions By A.D. Broadbent,Département de génie chimique, Université de Sherbrooke,Québec,Canada J1K 2R1 Description of the data obtened by Wright
the sums (integrals) are used to calculate by iteration or approximation all the terms of the Matrix M
a=.49
b=.31
c=.2
d=.17697
e=.8124
f=.01063
g=0
h=.01
i=.99
in 1931 WRIGHT ,GUILD and the CIE haven' t excell or open office calc
and used manual calculations but the results are the same.
try with excell calc or other tableur
try to read excell sheet from BROADBENT with GOOGLE
worksheets showing all calculations, **updated 21Aug09)
http://www.cis.rit.edu/mcsl/research/1931.php
Bercier (talk) 18:59, 14 November 2012 (UTC)
- You are nevertheless unintelligible. A couple of working links could help. Dicklyon (talk) 19:19, 14 November 2012 (UTC)
http://www.cis.rit.edu/mcsl/research/broadbent/CIE1931_RGB.pdf
Calculation from the original experimental data of the CIE 1931 RGB standard observer spectral chromaticity Co-ordinates and color matching functions By A.D. Broadbent,Département de génie chimique, Université de Sherbrooke,Québec,Canada J1K 2R1 Description of the data obtened by Wright
- Bercier: But I’ve been letting Dicklyon talk to you here, because I honestly can’t figure out what you’re trying to say, and therefore I have nothing to add. –jacobolus (t) 01:03, 15 November 2012 (UTC)
REMARQUE IMPORTANTE
[edit]
Soit:

Ces 3 intégrales permettent 3 formulations:
- 1°/Si λ2-λ1=dλ ==>0
alors:
Alors si :
et si :
- représentent les coordonnées d'un point situé sur le spectrum locus du GAMUT (le lieu du spectre) et correspondant à :λ=λ1=λ2.
POUR VOIR LES DOCUMENTS DE BROADBENT:[2]. worksheets showing all calculations, **updated 21Aug09)
VOIR BROADBENT page 10:
Ya, intensité lumineuse subjective indépendante de la couleur est la luminance qui peut être appelée la luminance absolue,d'où l'indice a.
Soit :
On calcule:
On mesure I(λ) avec le photométre à scintillements(flicker photometer)pour que les scintillements disparaissent en partant de λ=555 nm
On prend:
et:
Alors:
C'est le principe du calcul de V1924(λ) qui est inversement proportionnelle à la mesure de I(λ).
Since we want to plot a spectral sensitivity function derived by heterochromatic flicker photometry the dependent variable (radiance of the chromatic field that gave minimum flicker) has to be divided into 1.0. This gives us the reciprocal of the radiance which is the standard way of representing psychophysically derived sensitivity. When this reciprocal of radiance is plotted as a function of wavelength one has a photopic spectral sensitivity function.
Frederik IVES 1900.
- 2°/Si on considère 2 limites d'intégration λ1 et λ2:
alors si :
alors:
(xi,yi,zi) représentent les coordonnées d'un point intérieur au gamut situé entre les points représentant λ1 et λ2.
- 3°/Si cette intégration se fait de 360nm à 830nm .
alors λ1=360nm et λ2=830nm
Ces intégrations de 360nm à 830nm donnent une même valeur numérique disons N.
alors:
alors:
xW=yW=zW=N/3N=1/3
Les coordonnées du blanc sont (1/3,1/3,1/3)
CIE 1931-2° xyY: Y=0.17697r+0.81240g+0.01063b
Cette luminance peut être appelée la luminance (tout court)(voir Robert Sève).
Y/y=X/x=Z/z=X+Y+Z est appelée la luminance relative(voir Robert Sève).On peut ajouter barycentrique car elle permet de calculer le mélange des lumières.
LES FONCTIONS Y , Ys et Ya NE SONT PAS LES MËMES,
CE QUI REND TOUT LE TEXTE DE LA CONTRIBUTION de WIKIPEDIA CIE XYZ COMPLETEMENT INCOMPREHENSIBLE,
SI ON REPRESENTE CES 3 FONCTIONS PAR Y.
http://www.cis.rit.edu/mcsl/research/1931.php
texte:cliquer sur:CIE1931_RGB.pdf
tableur:cliquer sur:Cie1931_RGB_v2.xls
texte seulement:
http://www.cis.rit.edu/mcsl/research/broadbent/CIE1931_RGB.pdf
Calculation from the original experimental data of the
CIE 1931 RGB standard observer spectral chromaticity
Co-ordinates and color matching functions
Références
[edit]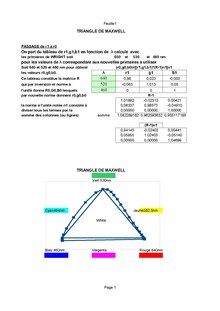
http://www.cis.rit.edu/mcsl/research/1931.php
- ^ Adobe (2005). "Adobe® RGB (1998)" (PDF). San Jose, CA: Adobe Systems Incorporated. AdobeRGB.
{{cite news}}: External link in|last1=|sous-titre=ignored (help) - ^ http://www.cis.rit.edu/mcsl/research/1931.php ource.com Titre de la source], sur le site de votre source. Consulté le « date du jour ».
1. ↑ A.RE-DETERMINATION OF THE TRICHROMATIC COEFFICIENTS OF THE SPECTRAL COLOURS BY W.D.WRIGHT MS,received,7th February,1929 Read and discussed,14th March ,1929. pages 141-164 dans Transactions of the Optical Society, vol. 30, no 4, 1er mars 1929
2.↑ A.RE-DETERMINATION OF THE MIXTURE CURVES OF THE SPECTRUM MS,received,3 1stJanuary,1930. Read and discussed,10th April,1930. pages 201-218
3.↑ http://www-cvrl.ucsd.edu/cmfs.htm CVRL Color & Vision database CIE (1931) 2 et 10 degré color matching functions λ xbar ybar zbar
4.↑ http://www.cis.rit.edu/mcsl/research/broadbent/CIE1931_RGB.pdf
Calculation from the original experimental data of the CIE 1931 RGB standard observer spectral chromaticity Co-ordinates and color matching functions By A.D. Broadbent,Département de génie chimique, Université de Sherbrooke,Québec,Canada J1K 2R1 Description of the data obtened by Wright
5.↑ http://sellig.sed.myriapyle.net/
Restitution formelle de la théorie sous-jacente à la norme colorimétrique XYZ CIE 1931 Calcul de la matrice de passage de RGB à XYZ par la résolution d'un systeme de 6 équations à 6 inconnues.
6.↑ http://www.photo-lovers.org/color.shtml.fr
Bercier (talk) 14:59, 23 March 2013 (UTC)
More discussion
[edit]My French and online translator are not good enough for me to quite understand the point. Apparently Bercier thinks the article is hash by not distinguishing Y, Ya, and Ys, whatever those are. If someone can help interpret, that might be useful. Dicklyon (talk) 19:01, 13 April 2013 (UTC)
Conflicting definitions of "tristimulus values"
[edit]In this revision (prior to my edits) "tristimulus values" are at first treated (apparently) as though they correspond to physical levels of stimulus of the three types of cone cell. This is the implication in the first three paragraphs of the "Tristimulus values" section, which use the term in relation to cones, the physical eye, and metamerism. This is also the implication of the word itself, which clearly suggests three kinds of physical stimuli.
Then, at the end of the fourth paragraph, the phrase "tristimulus values" is used in a radically different sense: now it relates to levels of imaginary primary colours that have only a very indirect relationship to actual stimulus in the eye. One of these "primary colours" is in fact luminance, which in physical terms can be increased by stimulation to any of the three cones.
I started rewording this section for readability by non-experts, but I'm now doubting whether my initial edits are correct. Please would someone confirm whether "tristimulus" relates to stimulus of the three types of cone, or whether it relates purely to the XYZ of the CIE space (and not directly to stimulus at all). Thanks, Fuzzypeg★ 23:39, 27 February 2013 (UTC)
- The “tristimulus values” of CIEXYZ are a purely physical quantity, the three weighted sums of a light source's intensities at various wavelengths, based on an artificially defined “standard observer”, not some real human’s cone responses. However, the standard observer is defined in such a way that two colors with the same tristimulus values should appear indistinguishable to some typical human, or that’s the goal anyway; in practice that’s not quite always perfectly true, as Grassman’s law is only a model, and there are variations in perception from one observer to another, and even within the same observer depending on other factors. –jacobolus (t) 03:29, 1 March 2013 (UTC)
- So you seem to be saying that the tristimulus values (XYZ) are analogous to the stimuli to LMS cones in an artificially defined "standard observer". That seems eminently sensible, and appears supported by some of the diagrams. But it is contradicted by the following:
- "Y means luminance, Z is quasi-equal to blue stimulation, or the S cone response, and X is a mix (a linear combination) of cone response curves chosen to be nonnegative."
- If Y is luminance, then that implies that Y weights all wavelengths evenly, which doesn't appear to be the case in the diagrams. Is the quoted sentence simply incorrect? Or am I missing something? Thanks, Fuzzypeg★ 12:11, 17 March 2013 (UTC)
- So you seem to be saying that the tristimulus values (XYZ) are analogous to the stimuli to LMS cones in an artificially defined "standard observer". That seems eminently sensible, and appears supported by some of the diagrams. But it is contradicted by the following:


- I've figured it out! is the photopic luminosity function, which just happens to be quite similar to a green response curve. I had expected luminosity to be a much flatter function across most of the visible range, trailing off only toward the very edges of the visible range. It just didn't look like luminosity to me — it looked like a green response curve! Cheers, Fuzzypeg★ 22:01, 17 March 2013 (UTC)
- The Y (luminance) curve is EXACTLY the "green" curve of the XYZ color matching functions, by definition and construction. Don't think of it as green and you'll be better off. Dicklyon (talk) 14:44, 10 April 2013 (UTC)
see
http://www.cis.rit.edu/mcsl/research/1931.php
Ya whole is ABSOLUTE Luminance [ edit ]Ya is one indefined integral but really one summation by 5 nm to 5nm between the extremities of the visible spectrum like tabulated by V1924=ybar by the CIE 1931-2° also 360nm to 830nm.
For CIE xyY 1931-2° Ya is for calculation
1/if you take a ray of wawelenght λ
and for the equi-energetic spectre S(λ)=S
O = xbar * S
also y = O / (XA + Ya + Za)
we have also y=Y/(X+Y+Z)
but Y( LUMINANCE ) is different of Ya:a for ABSOLUTE LUMINANCE
2/For all the spectrum from 360nm to 830nm
and for the equi-energetic spectre S(λ)
Ya is the area under the curves of the integral and the CIE 1931xyY-2° choose Xa=Ya=Za
3/ You have also:
Ra=Ga=Ba
r=Ra/(Ra+Ga+Ba)=g=b=1/3
4/To return to WRIGHT's(10) and GUILD's(7) experimentations
we have to inverse the matrix Mt
(rbar,gbar,bbar)=(xbar,ybar,zbar)*Mt-1
rbar=2,3635xbar -,5151ybar +,0052zbar
gbar=-,8958xbar +1,4264ybar -,0145zbar
bbar=-,4677xbar +,0887ybar +1,0093zbar
sbar=rbar+gbar+bbar
r=rbar/sbar
g=gbar/sbar
b=bbar/sbar
r,g,b are the calculed WRIGHT+GUILD'results with 2nm differencie with the WRIGHT+GUILD 's real experimentations(10+7) 1930.
5/we can re-start with r,g,b
Y is determined so that the areas under the curves rbar, gbar bbar and are equal to each other and equal to the surface as V1924. either Ra = Ga = Ba
or D = dr + eg + fb is an identity
In calculant:
(Rbar, gbar, bbar) = (r, g, b) * V1924 / D is found by successive adjustments equivalent to the least squares method or the method of successive approximations or Newton iterative calculation:
So there is
d = 0.17697 e = 0.8124 f = 0.01063
This determines Y = +0.8124 0.17697r g+ 0.01063b LUMINANCE 1/0.17697 a coefficient = 5.65 (08) around which does not play on the various calculations.
Then writing that xbar and zbar (ie x and z) have no negative value and the graph y (x) is tangent to the axes found by successive adjustments
en calculant xbar=arbar+bgbar+cbbar et zbar=grbar+hgbar+ibbar:
a=0.49 b=0.31 c=0.2
g = 0 = h = 0.01 to 0.99
thereby calculate
X = 0.49r +0.31 D 0.2b et
Z = 0.01g +0.99 b
and therefore the relative barycentric luminance L = X + Y + Z which allows to determine the color gamut with the mixture of n lights as barycenter points x, y, z of their assigned weight relative luminance Ln.
all these calculations are very easy with Libre Office tableur
reference:BROADBENT
Bercier (talk) 12:33, 6 February 2013 (UTC)
Bercier (talk) 09:46, 1 June 2013 (UTC)
CIE etc. edits by Physicsxuxiao
[edit]The following words are from Dicklyon:
You can post questions on the article talk page, instead of on your user page, and be more likely to be noticed.
Please review your edits before saving, make sure the sentences are readable, and add spaces after periods and commas. I'm working on cleaning up your recent contributions. Dicklyon (talk) 16:02, 27 June 2013 (UTC)
I took out your main new paragraph, as it's unsourced and hard to interpret, and I think misattributes the finding that no set of real primaries can span all colors; didn't Maxwell have that already? Here it is, for reference:
- In early color vision studies, sensitivity peak response of the three types of cone cells were thought to correspond with three kinds of light wave bandwidths with fairly narrow range, these generally correspond to red, green and blue light. Thus, tristimulus values associated with a color space called RGB color spaces were first used to describe this behaviour of the cone cell types. These three colors comprised the threeprimary colors in a tri-chromatic additive color model. But in the early 1900's experiments of W. David Wright[3] and John Guild[4] proved that it was in fact impossible for the human eye to sense some colors unless some values of tristimulus values are negtive. Therefore, the three primary colors in a CIE XYZ description for color space were not adequate or optimum descriptions for real colors in the sense negative values for tristimulus values were needed to describe colors sensed by the eye, and this capable CIE XYZ model could be improved with some revisions. The following better explains CIE XYZ and revisions made to improve modeling human color vision.
What you're trying to do seems OK, but let's base it on sources and write it carefully. Respond here if you have a source that you're relying on, and I'll look at it, too. Dicklyon (talk) 16:15, 27 June 2013 (UTC)
The following words are from physicsxuxiao:
Thank you for your help,I revised this paragraph with my memory of the text book by Hunter,who established the CIELAB space.But my book has been lost.Yet you can find some clues in Young–Helmholtz theory and the experiments of W.David Wright[3] and John Guild[4]. And the idea of analyzing mixing color by mathmatics is from Hermann Grassmann,so I don't think Maxwell know that no set of real primaries can span all colors.The story of primary colors must complemented by the experiments of W.David Wright[3] and John Guild[4].
Retrieved from "http://en.wikipedia.org/w/index.php?title=User_talk:Physicsxuxiao&oldid=561850618"
Physicsxuxiao (talk) 17:58, 27 June 2013 (UTC)
- This 1927 printing of a 1915 book discusses the Maxwell color triangle and notes that "It is thus seen that spectral colors throughout a large range of wave-lengths arouse the three primary sensations, according to the Young–Helmholtz theory." From his color triangle sketch and interpretations such as these, it always seemed clear to me that Maxwell was the one who pinned down the way the tri-chromatic theory really works, before Wright and Guild did their more careful measurements. Dicklyon (talk) 18:39, 27 June 2013 (UTC)
- what I mean is: (a)The tri-chromatic theory, evoluted from the ideas of Young,Helmholz and Maxell,maybe including Grassmann, really works; (b)But we know that 'no set of real primaries can span all colors.'by the experiments of Wright[3] and John Guild[4].Physicsxuxiao (talk) 05:16, 28 June 2013 (UTC)
- I can't read that book you pointed out.But just by that "It is thus seen that spectral colors throughout a large range of wave-lengths arouse the three primary sensations, according to the Young–Helmholtz theory.", I can't make a conclusion that Maxwell has known the problem.Physicsxuxiao (talk) 14:19, 28 June 2013 (UTC)
- No, you certainly can't conclude that from that snippet. But you can certainly conclude that it known before Guild and Wright's experiments. It's also discussed in William de Wiveleslie Abney's 1891 book on colour measurement and mixture; he shows "Koening's Curves of Colour Sensation" greatly overlapping, using an apparatus like Maxwell's but doing a better job of it. Dicklyon (talk) 22:52, 28 June 2013 (UTC)
- I can't read this book because there is no e-book version at book on colour measurement and mixture.However,I believe what you said by now now that you have so many proofs.Physicsxuxiao (talk) 01:30, 29 June 2013 (UTC)
- No, you certainly can't conclude that from that snippet. But you can certainly conclude that it known before Guild and Wright's experiments. It's also discussed in William de Wiveleslie Abney's 1891 book on colour measurement and mixture; he shows "Koening's Curves of Colour Sensation" greatly overlapping, using an apparatus like Maxwell's but doing a better job of it. Dicklyon (talk) 22:52, 28 June 2013 (UTC)
Old Version and it's Change on july 7th,2013,
Old Version:
A color space maps a range of physically produced colors (from mixed light, pigments, etc.) to an objective description of color sensations registered in the eye, typically in terms of tristimulus values, but not usually in the LMS space defined by the cone spectral sensitivities. The tristimulus values associated with a color space can be conceptualized as amounts of three primary colors in a tri-chromatic additive color model, although in most cases, such as the LMS space and the CIE XYZ space, the primary colors used are not real colors, in the sense that no nonnegative light spectrum can produce those colors.
New Editing:
A color space maps a range of physically produced colors (from mixed light, pigments, etc.) to an objective description of color sensations registered in the eye, typically in terms of tristimulus values, but not usually in the LMS space defined by the cone spectral sensitivities. The tristimulus values associated with a color space can be calculated as amounts of three primary colors in a tri-chromatic additive color model by the way of color triangle, with an arrangement of colors within a triangle, based on the additive combination of three primary colors at the triangle's corners.In most cases, such as the LMS space and the CIE XYZ space, in order to comprise all the colors that we can sense, the primary colors used are not real colors, in the sense those colors can be noted in the color space mathematically but not be sensed by our eyes. Physicsxuxiao (talk) 15:24, 24 July 2013 (UTC)
- It might be better than you had before, but still not quite right. "The tristimulus values associated with a color space can be calculated as amounts of three primary colors in a tri-chromatic additive color model by the way of color triangle, with an arrangement of colors within a triangle, based on the additive combination of three primary colors at the triangle's corners" is pretty uninterpretable. And the final "but not be sensed by our eyes" is quite wrong; the limitation is not with our eyes, but with the impossible physics of negative spectral powers. Dicklyon (talk) 07:34, 25 July 2013 (UTC)
- No,"the primary colors used are not real colors, in the sense that no nonnegative light spectrum can produce those colors."is absolutely wrong.In fact,according to the experiments of W.David Wright[3] and John Guild[4],those colors with 'negtive spectral powers' as your words may be real,just not be in the color triangle while the three primary colors has been selected.But if you want to mix up all the real colors with Grassmann's Law, you have to choose a set of 'fraud' primary colors which means they can't be seen by human eyes.Physicsxuxiao (talk) 14:44, 26 July 2013 (UTC)
- Dear Dicklyon, I've changed the paragraph back.I'm sorry for the edit war.I will give more evidence later.Physicsxuxiao (talk) 16:14, 26 July 2013 (UTC)
- The colors of all nonnegative spectra are in "the tongue-shaped or horseshoe-shaped figure shown in color". Primaries that make a triangle that includes that shape in its interior must necessarily have corners outside that region, and they therefore do not correspond to real colors, or to light spectra. I do not understand the point you are trying to make above (partly because I don't follow your English, like what does "just not be in the color triangle while the three primary colors has been selected" refer to, and what does it mean? And what do you mean by "can't be seen by human eyes"? Can you describe the spectrum of such a color?) Let's work out the wording here instead of inserting errors into the article. Dicklyon (talk) 23:07, 26 July 2013 (UTC)
- In different color space, a color has different tristimulus values and some 'real' color has negtive conponents for it's tristimulus values.You can find these kinds of colors in RGB space.In addition,I don't kknow what you mean when you use the phrase 'nonnegtive spectra'.Therefore, I can't understand the sentence, "the primary colors used are not real colors, in the sense that no nonnegative light spectrum can produce those colors."
- In fact, I don't think the explelation of tristimulus values is readable and accurate.Maybe I should creat another article.Physicsxuxiao (talk) 02:03, 27 July 2013 (UTC)
- We are in agreement on the point that in different color spaces, a color has different tristimulus values, and some 'real' colors have negative conponents for their tristimulus values. When I speak of a nonnegative spectrum, I mean a spectrum of light that might possibly exist physically, with some amount of light at every wavelength, but without negative amounts; every such spectrum is either invisible (black) or has a real color. As to the rest, I will wait and see if some other editor will comment or clarify. Dicklyon (talk) 02:49, 27 July 2013 (UTC)
- Yes, we both know what we are talking about.But nonnegative spectrum is a very technical phrase and the phrase should be used on a special color space.Therefor,I think that the utility of the phrase here is not suitable. On the other hand, avoiding edit war, my suggestion is, "some of primary colors used not real colors, in the sense that they can't be generated with any kind of light spectrum."Physicsxuxiao (talk) 15:31, 27 July 2013 (UTC)
- Another problem is that tristimulus values,which is from psychological theory,"stimulus and reaction",based on that we have three cones,can't be used to discribe all the parameters in any kind of color spaces.For example, we think that discribing the HSV color space with tristimulus values is unsuitable.Physicsxuxiao (talk) 15:45, 27 July 2013 (UTC)
- OK, we can use "In some color spaces, the primary colors used are not real colors, in the sense that they cannot be generated with any light spectrum." And please use spaces after your periods and commas to make your text more normal and readable. I'm not sure what you're saying about "stimulus and reaction", or in what sense tristimulus values are "unsuitable"; they are a quite different from an HSV description; nonlinearly related; does that mean "unsuitable"? Dicklyon (talk) 16:50, 27 July 2013 (UTC)
- We are in agreement on the point that in different color spaces, a color has different tristimulus values, and some 'real' colors have negative conponents for their tristimulus values. When I speak of a nonnegative spectrum, I mean a spectrum of light that might possibly exist physically, with some amount of light at every wavelength, but without negative amounts; every such spectrum is either invisible (black) or has a real color. As to the rest, I will wait and see if some other editor will comment or clarify. Dicklyon (talk) 02:49, 27 July 2013 (UTC)
- The colors of all nonnegative spectra are in "the tongue-shaped or horseshoe-shaped figure shown in color". Primaries that make a triangle that includes that shape in its interior must necessarily have corners outside that region, and they therefore do not correspond to real colors, or to light spectra. I do not understand the point you are trying to make above (partly because I don't follow your English, like what does "just not be in the color triangle while the three primary colors has been selected" refer to, and what does it mean? And what do you mean by "can't be seen by human eyes"? Can you describe the spectrum of such a color?) Let's work out the wording here instead of inserting errors into the article. Dicklyon (talk) 23:07, 26 July 2013 (UTC)
2 degrees angular radius or diameter?
[edit]There is an important information missing: Does the 2-degree figure refer to the angular radius (i.e. up to 2 degrees off the central visual axis) or the diameter (i.e. 2 degrees rim to rim)? Even the freely available sources cited here do not clarify this. Or is there some convention how fields of view are specified?--SiriusB (talk) 19:56, 29 July 2013 (UTC)
it's diameter,not radius.See WRIGHT. Bercier (talk) 08:33, 30 July 2013 (UTC)
- Thanks. This should be written explicitely in the article since it is far from being self-explanatory (normally, the radius is used where an approximate radial or axial symmetry is present). I have added it now. The Wright paper is copyright-censored and not available to the library account of my institute, so I cannot look up (unless you refer to another paper than the one the 1931 standard is based on).--SiriusB (talk) 09:50, 30 July 2013 (UTC)
- Addition: I've found a direct link to the paper. Maybe the journal website was broken when I tried, but not it seems to work again. It would have been a surprise to have a 80 years old paper behind a paywall... So, diameter instead of radius seems to be more widely used in the early 20th century, and since CIE 1931 is a standard (and a starting point for new ones) this has been continued up to now.--SiriusB (talk) 12:59, 30 July 2013 (UTC)
- Diameter is a convention for optical engineering,see field of view or angle of view.Physicsxuxiao (talk) 15:55, 1 August 2013 (UTC)
Inverse of matrix
[edit]User:Dicklyon has reverted my edit of the other day, in which I added the inverse of the matrix for going from RGB to XYZ. Dicklyon wrote as a comment: "this matrix woud need a source; it does not appear to be approximately right even". Well, as I wrote in my edit comment, I took it from the French version. The French version gives three references at that point -- unfortunately one is a book, one is a pdf you have to buy (!), and one seems to be only a blurb about some official manual. But I did check whether the matrix in the French version was the inverse (using Excel), and I get practically the same result! For example, instead of 0.418456 I find 0.418466, and instead of 0.252426 I get 0.252431. I don't know why there are these tiny differences, but that's not enough of a discrepancy to revert my edit! (I do notice now that the formula which I used from the French uses commas instead of periods in the numbers -- that should be changed.) Eric Kvaalen (talk) 06:54, 27 August 2013 (UTC)
- Yes, I looked at the French version, too. Its sources are inaccessible and unclear. It was recently modified in this edit to more nearly match what we have in English, but that left it in an inconsistent state, both typographically a mess and mathematically imprecise. Those numbers should be checked, sourced, and corrected, not copied into en. I may have been wrong about it being approximately right; I didn't check, but found a book that seemed to show a matrix about like that being the inverse of the other one without its leading factor; that was wrong. Dicklyon (talk) 20:40, 27 August 2013 (UTC)
- Matlab suggests these numbers (long double format):
0.418465712421894 -0.158660784803799 -0.082834927618095 -0.091168963909023 0.252431442139465 0.015707521769558 0.000920898625344 -0.002549812546863 0.178598913921520
- But I'd still rather see something sourced. Dicklyon (talk) 21:01, 27 August 2013 (UTC)
- I don't think we need a reference for inverting a matrix! I'm putting the inverse back in. I'll use your calculation (which agrees with Excel), rounded to five digits. Eric Kvaalen (talk) 14:36, 30 August 2013 (UTC)
- What's the point of including the inverse matrix, exactly? No one uses the chromaticity coordinates in this particular RGB model anymore, so the only real interest in including the matrix in the forward direction is for explaining the historical development of the CIE system. The inverse matrix doesn’t really add anything, and anyone who needs it for some niche purpose can trivially calculate their own. –jacobolus (t) 09:14, 31 August 2013 (UTC)
Are all colours outside the triangle impossible on an RGB screen then?
[edit]So where Greeen, #00FF00 is, there is no color after that at the top in the grey area? Then how come in a different image with some other bluish green there it is visible, should that image be removed? — Preceding unsigned comment added by 88.104.101.221 (talk) 16:43, 18 October 2013 (UTC)
Proposed merge with Tristimulus timbre model
[edit]This seems to be a subset of what is already on the other page. It should be over there. But I'm not an expert. 🍺 Antiqueight confer 12:44, 21 October 2013 (UTC)
- Oppose – Why do you think a model of audio stimuli should be moved into an article about color? I don't get it. –jacobolus (t) 06:02, 22 October 2013 (UTC)
- I thought I pulled the transfer - I thought I had rolled that back once I read it again. Obviously I missed it here.-- 🍺 Antiqueight confer 10:35, 22 October 2013 (UTC)
Bit depth?
[edit]Rec. 709 has a bit depth of 8 bits per RGB channel, for a total of 24 bits of color. while Rec. 2020 has a bit depth of 12 bits per channel for a total of 36 bits of RGB color. My question is, how many bits per channel would it take to encode the entire CIE 1931/XYZ color space? Bumblebritches57 (talk) 01:48, 6 December 2013 (UTC)
As the CIE space is defined in real numbers, thus you may use as many more bits to code them as you want. For the bit depth the usual argument is that at 12 bits per color humans can usually not discriminate between steps on the scale,however this is not defined in any way in the CIE space.
XYZ relationship to LMS?
[edit]the page doesn't define "quasi" blue of Z tristimulus value, I think, it says quasi-blue but doesn't say where or how Z comes about, is the RGB just LMS that is close to an RGB or the RGB of LMS?
the same question for X tristimulus value, is it really just a combination of LMS responses close to RGB, or the RGB of LMS?
the page is not clear of xbar,ybar,zbar derivation, I think
are xbar,ybar,zbar defined to obtain XYZ tristimulus values? the page does not say one way or another as far as I comprehend
is there a device definition of xbar,ybar,zbar like the page indicates for rbar,gbar,bbar (mercury vapor?)? Dale r kelly (talk) 03:30, 11 January 2015 (UTC)
- Dale, the X, Y, and Z are linear combinations of L, M, S. That is they are different bases for the same 3D subspace of spectral space. I think some of the refs explain the derivation. Basically, iirc, the Y is first defined to correlate well with luminance. Then the Z is defined as a compact non-negative blue peak, and then X gets what's left, transform to be as compact as possible while staying nonnegative. Or something like that. So Z is not too far from S. Dicklyon (talk) 03:42, 11 January 2015 (UTC)
Thanks. The derivation of XYZ from Wright-Guild data could be more clear in terms of what comes first,
whether that be XYZ or xbar,ybar,zbar, or whether these play together in based on commonality.
color matching functions are defined as chromatic sensitivities of the eye, whereas LMS is defined as the absolute sensitivity of the eye, I know what chromaticities are, does the LMS cone reponse mix in "some" luminousity as well as chromaticity?Dale r kelly (talk) 04:13, 13 January 2015 (UTC)
xbar,ybar,zbar have a luminousity correlate rbar,gbar,bbar have a emprirical correlate other than the eye? mercury vapors?
RGB to XYZ conversion (and vice versa) error?
[edit]I am currently working on a script which includes conversion from RGV otXYZ and vice versa, but when I used the data from the matrix, I recived abosoluty wrong results when converting a rainbow back and forth; but the data from cs.rit.edu yielded much better results. Is this acctually a problem with the Matrix [2] or was I using the data for the wrong task? And if not, shouldn't the (correct) conversion matrix be added to the wiki?
--Surviavi (talk) 22:43, 7 September 2015 (UTC)
- Can you elaborate about what the difference between matrices was, exactly what you tried, and what issue you ran into? Note that this matrix you linked to in the Wikipedia page is not for sRGB primaries, but for the RGB primaries from the original Wright/Guild data. It’s not meant to be used to convert colors for the web, for example. If you want a matrix for sRGB colors, you should look at sRGB#Specification of the transformation. –jacobolus (t) 07:37, 11 September 2015 (UTC)
- The matrices are only a conversion aid, and the RGB->XYZ works somewhat better. Values with ranges from [0-1.0]. Anything outside of that is outside the visible spectrum. In addition, to use those tables the R/G/B values first must be divided by MAX_INT for whatever size you're using, run through a conditional check to handle smaller values differently... looks like this in Go:
if r > 0.04045 {
r = pow32((r + 0.055) / 1.055, 2.4)
} else {
r = r / 12.92
}
Finally that gets multiplied by 100, run through the matrix, clamped to your color bit-width if going to RGB, and returned.
Apparent error in the text of the page:
[edit]In the first sentence of the text, under "Tristimulus values", I find "short (S, 420–440 nm)". Just to the right of this text is a diagram that clearly shows the short peak at about 450 nm. These cannot both be correct. The diagram appears to be good; if so, the peak should be stated to be 440-460 nm rather than 420-440. I am reluctant to edit the page, but this is clearly a real issue. Jonsinger (talk) 21:58, 13 November 2015 (UTC)
Lightness of chromaticity diagrams?
[edit]The xy chromaticity diagrams in this article do not tell you the value for Y which they used. This should really be mentioned in the image captions. SharkD Talk 16:38, 26 November 2016 (UTC)
- Having done many of these kinds of renderings I can tell you that the brightness they used is not uniform in the image. It is instead the brightest possible Y value for each pixel. Near the white point that will be Y = 100 and elsewhere it is lower.
I have considered replacing this with another style image. Maybe someday I'll get around to it. TDcolor (talk) 03:22, 5 January 2021 (UTC)
Construction of the CIE XYZ color space from the Wright–Guild data
[edit]Where does the 1 / b21 originate from because it's not from the cited paper?
I have no clue either. Searched for hours where this comes from. Cannot find it anywhere (not a single article, or standard). — Preceding unsigned comment added by 2A02:A03F:E40F:7C00:6156:16F8:3715:683C (talk) 11:05, 15 December 2020 (UTC)
This matter is discussed in https://onlinelibrary.wiley.com/doi/10.1002/(SICI)1520-6378(199710)22:5%3C335::AID-COL7%3E3.0.CO;2-Y and in https://onlinelibrary.wiley.com/doi/epdf/10.1002/%28SICI%291520-6378%28199808%2923%3A4%3C259%3A%3AAID-COL18%3E3.0.CO%3B2-7 After having read these, I think the normalization should be dropped indeed. This normalization is actually the result of how the best-fit coefficients to obtain V(lambda) from r, g, b functions was influenced by the fact that r(lambda) was scaled such that r(700nm) = V(700nm). The standard matrix as specified by CIE should not contain this normalization, as it is balanced for the Equal Energy illuminant. — Preceding unsigned comment added by 212.224.226.15 (talk) 14:09, 25 March 2022 (UTC)
- Dropping the normalization value is indeed correct. It is used when transforming the color matching functions r[hat](lambda). g[hat](lambda) and b[hat](lamda) to x[hat](lambda), y[hat](lamda) z[hat](lambda). This constant arises from the fact that the areas under the r,g,b tristimulus functions were less than the area under the photopic response curve by the factor of 0.17697. However, when R, G and B are calculated from the r,g,b color matching function, that factor is implicitly incorporated in order for a perfect diffuse reflector of illuminant E to have R, G and B values of 100, 100, 100. From what I can gather, the photopic response curve was scaled so that its maximum value is 1.0 and the r,g,b color matching functions are scaled so that they all have the same area and so that the r color matching function at 600 nm was equal in value to the photopic response curve at that wavelength. No idea why they did this rather than have the areas under all four curves (photopic response and the r,g,b color matching functions) equal. Of course, when XYZ color space was developed, Y was set equal to the photopic response curve, so it of course has the same area. X and Z are then scaled to also have that area. I had noticed that the matrix that had been shown with the (1/0.17697) factor was wrong for a while, but am new to Wikipedia and didn't know how to correct it. I am glad someone beat me to this! 97.78.195.34 (talk) 01:38, 8 April 2022 (UTC)
- Oops - meant the values at 700 nm, not 600. 97.78.195.34 (talk) 01:39, 8 April 2022 (UTC)
Meaning of X, Y and Z .. please!
[edit]Wiki: "The unit of the tristimulus values X, Y, and Z is often arbitrarily chosen so that Y = 1 or Y = 100 is the brightest white that a color display supports . The corresponding whitepoint values for X and Z can then be inferred using the standard illuminants."
So in 1931 when CIE defined all these, a "color displays" was in question?
Please stay serious! — Preceding unsigned comment added by 62.202.181.251 (talk) 02:57, 16 February 2018 (UTC) CMon, sorry it is only s**t, better delete it. Refer to 1931 and mention at the same time "color display support", who will take you as a serious source?
Ok, reverted once again. I hope you are happy and nobody ask you the relation between Y and a "color display" - which seems to be the basic for you wiki's. *lol* explaining CIE-Y (1931) in relation with "color display" explains the wiki "competence" :( — Preceding unsigned comment added by 62.202.181.251 (talk) 03:32, 16 February 2018 (UTC)
- We could discuss that here, but if you remove the remarks of others it's not going to go anywhere. Let me know if you'd like to discuss. Dicklyon (talk) 23:03, 16 February 2018 (UTC)
Requested move 11 August 2018
[edit]- The following is a closed discussion of a requested move. Please do not modify it. Subsequent comments should be made in a new section on the talk page. Editors desiring to contest the closing decision should consider a move review. No further edits should be made to this section.
The result of the move request was: Not moved. (non-admin closure) The Duke of NonsenseWhat is necessary for thee? 20:12, 18 August 2018 (UTC)
CIE 1931 color space → CIE 1931 color spaces – Plural. There are more than one of these color spaces with different names. See the lead paragraph of the article. SharkD ☎ 23:01, 11 August 2018 (UTC)
- Oppose – The singular makes perfect sense even though there are several such color spaces discussed in the article; so per WP:PLURAL, keep it singular. Dicklyon (talk) 05:50, 12 August 2018 (UTC)
- Per WP:PLURAL: "There are two main types of exceptions to this rule: 1. Articles on groups or classes of specific things..." OTOH, I did count, and the singular is used more often than not in the article. SharkD ☎ 07:30, 12 August 2018 (UTC)
- Oppose Keep it singular per WP:PLURAL--these color spaces are not almost always discussed as a set, as with a topic like Maxwell's equations; rather there are simply two of them. Same with the more general color space. --
{{u|Mark viking}} {Talk}11:31, 12 August 2018 (UTC)
- Actually, according to the article there are four: CIE XYZ, CIE xyY, CIE RGB, and CIE rg. (I searched on the Web and could not find any instances of CIE rgY, though one could certainly create this color space if one wanted to invent a new one.) SharkD ☎ 13:35, 13 August 2018 (UTC)
- The above discussion is preserved as an archive of a requested move. Please do not modify it. Subsequent comments should be made in a new section on this talk page or in a move review. No further edits should be made to this section.
Remove unreferenced section for CIE xy chromaticity diagram and the CIE xyY color space
[edit]I added sources for the equations in the section. This was no big deal. I wonder why this was not done ages ago. My question: did I miss something or is it time to remove the "unreferenced" tag? AndreAdrian (talk) 10:10, 11 December 2018 (UTC)
- I think it should go, especially since it says "not....any sources". But the section is still light on sourcing. North8000 (talk) 11:51, 11 December 2018 (UTC)
Remove code in § Color matching functions?
[edit]CIE 1931 color space#Color matching functions describes an approximation of the color matching functions of terms of elementary functions, and provides a definition of this approximation by way of a Haskell implementation. I have edited this section so that this definition is now given in a mathematical fashion, and have also added a C implementation. Given that a mathematical definition is now present, should the Haskell and/or C implementations remain in the article? — JivanP (talk) 00:38, 10 April 2020 (UTC)
- Yes - keep at least the C code. Why not. Currently one cannot even cut and paste the equation coefficients since they are presented a a PNG or SVG. MxBuck (talk) 03:34, 27 June 2024 (UTC)
Error in Gaussian Equations
[edit]Apparently, all arguments of the Gaussians, which describe the x, y, z color matching function, except the first argument should have an additional point/comma. For example, the Gaussians should not be centered around 5998, but 599.8, same is true for the sigma 1 and sigma 2 values. This can be easily seen from the linked paper which is publicly available.
Feel free to check this error yourself and, if you come to the same conclusion, potentially change it. — Preceding unsigned comment added by 91.19.232.210 (talk) 13:59, 18 June 2020 (UTC)
- The equations use angstroms as the unit, 10 angstroms = 1nm so these equations seem correct to me. TDcolor (talk) 02:33, 18 October 2021 (UTC)
- It looks like the unit choice here was to eliminate fractions so the code could be written as integers. For a Wikipedia article, it would probably be clearest to just use mathematical notation instead of a code implementation, and use nm as a unit. –jacobolus (t) 16:01, 18 October 2021 (UTC)
How can Y represent luminance if y chromacity coordinate changes in xyY with a change of the Y coordinate
[edit]y is defined as . Thus Y cannot represent luminance, cause the y coordinate changes if Y changes. Only if we set X = Z = 0, we get a fixed y coordinate in the xy-chromacity diagram. But as soon as X or Z have a non zero value, the y chromacity coordinate changes if you change Y. This is a contradiction. --95.91.242.234 (talk) 10:07, 23 May 2022 (UTC)
- @95.91.242.234 Something is completely wrong here. I think Z represents luminosity, but x and y, as represented in the article, don't make sense to me either. 92.252.104.29 (talk) 04:47, 19 October 2023 (UTC)
- @92.252.104.29 To correct myself. I just don't know what's going on. 92.252.104.29 (talk) 05:01, 19 October 2023 (UTC)
- Y can represent luminance, while still changing the x, y chromaticity coordinates. The reason is that luminance is mostly determined by the amount of green light. Red contributes only a little bit to luminance and blue contributes almost nothing at all. So to increase the luminance, you must add green. Unless you also add red and blue, the hue will of course change. XYZ is designed so that adding X automatically subtracts from green so that luminance stays the same. MTres19 (talk) 21:41, 24 April 2024 (UTC)
Meaningful, imaginary colors in XYZ
[edit]"In XYZ space, all combinations of non-negative coordinates are meaningful, but many, such as the primary locations [1, 0, 0], [0, 1, 0], and [0, 0, 1], correspond to imaginary colors outside the space of possible LMS coordinates; imaginary colors do not correspond to any spectral distribution of wavelengths and therefore have no physical reality." Firsly what does "meaningful" constitute in the first sentence? Secondly, "possible LMS coordinates", I assume means "subspace of valid LMS coordinates that can can be achieved in a human with normal eyesight", but it could also be taken to mean invalid LMS coordinates like negative values for a component. If I understand correctly, it is currently only assumed that the CrCgCb triangle is contained within whatever space valid LMS occupies in the rg diagram. If it's the case that the valid XYZ space contains invalid LMS values, then "meaningful" in the first sentence doesn't seem right at all. I'm new to this so tell me if I got it all wrong.
p.s. I used "subspace" in the colloquial sense, it very well might not meet the mathematical definition. TheOnlyRealEditor (talk) 08:00, 19 August 2022 (UTC)
Discrepancy between LMS --> XYZ coordinates from the HPE matrix?
[edit]When I tried converting the normalized LMS color cone response to CIE 1931 XYZ, there seemed to be a big discrepancy from the S cone response to the Zbar graph. Supposedly, the Zbar should only consist of the S cone response, which tops out at 1. The matrix also does not scale the S cone response by any amount. However, the actual Zbar function's maximum is approximately 1.78 at 445nm . Have I made a mistake somewhere? 66.183.66.205 (talk) 07:14, 19 January 2024 (UTC)
Regarding Section "CIE xy chromaticity diagram and the CIE xyY color space"
[edit]Since the gamut is defined by the projection of X,Y,Z onto the x,y plane , it becomes a triangle, which is intrinsically convex. So the discussion of convexity is unnecessary and just adds confusion, unless one adds more sensor bands or channels as is the case in multi-spectral satellites and spectroscopy. Then the mult-stimuli projection should be convex.
Lets skip this complication for the tristimlulus and RGB cases.

































































































